Linear momentum problem with n particles

[hquang001]

Homework Statement

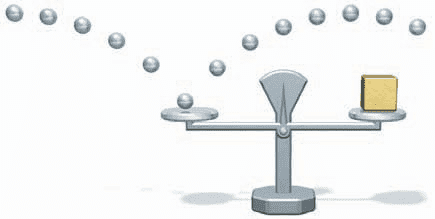
A stream of elastic glass beads, each with a mass of 0.50 g, comes out of a horizontal tube at a rate of 100 per second. The beads fall a distance of to a balance pan and bounce back to their original height. How much mass must be placed in the other pan of the balance to keep the pointer at zero?

Relevant Equations

$$F = \displaystyle{\frac{\bigtriangleup{p}}{\bigtriangleup{t}}} = n.m.v$$

$$KE_{initial} + PE_{initial} = KE_{final} + PE_{final}$$

To find the mass in other pan, i need to find the force caused by beads on the pan
∴ KEinitial + PEinitial = KEfinal + PEfinal
0 + mgh = ½ mv^2
=> v = 3.13 m/s

∴ The change in momentum :
p2 - p1 = m ( v2-v1) = m( v - (-v)) = 2mv

∴ F = Δp / Δt = n. m. v
How can i apply the rate of 100 per second in this equation ?

 

[haruspex]

F = Δp / Δt = n. m. v

What is n in that equation? What dimension must it have to make the equation consistent?

 

[hquang001]

What is n in that equation? What dimension must it have to make the equation consistent?

n is the number of particles or in this case glass beads and its unit is 1/s i think ?

and so i think 100/s = 1/ Δt => Δt = 1s/100

=> F = 0.313 N
=> M = 0.313/ 9.81 = 31.9 g

 

[haruspex]

n is the number of particles or in this case glass beads and its unit is 1/s i think ?

If n is just a number of beads, how can it have units 1/s? It must the number of beads per some period of time.

=> F = 0.313 N

How do you get that? Please show your steps,

 

[hquang001]

If n is just a number of beads, how can it have units 1/s? It must the number of beads per some period of time.

I'm not sure about it, but as i read, n is number of beads.

I think because F = n.m.v
F has SI units of kg.m/s^2
on the right side, m.v is [kg.m/s] so i think n should be 1/s so that it has equal unit to force ?
or is it number of beads per second ?

How do you get that? Please show your steps,

F = Δp / Δt
As i said above, 100/s = 1/ Δt => Δt = 1s/100

so F= Δp / Δt = (2 x 0.0005 x 3.13 ) / (1/100) = 0.313N

the force on this side must be equal to the other pan
so F = M.g => M = 31.9g

 

[haruspex]

is it number of beads per second ?

Yes.

so F= Δp / Δt = (2 x 0.0005 x 3.13 ) / (1/100) = 0.313N

Ok, that is right. I was thrown by not having picked up an earlier error. Given your calculation of v, you should have written F=nm(2v).

 

[hquang001]

Ok, that is right. I was thrown by not having picked up an earlier error. Given your calculation of v, you should have written F=nm(2v).

Oh if so "v" in n.m.v is the change in velocityi just showed the full formula with the part n.m.v but didn't know how to deal with this part so i used instead Δp / Δt

 

[haruspex]

Oh if so "v" in n.m.v is the change in velocity

Yes.

 

[kuruman]

Is the "original height" to which the beads bounce back given some place and I missed it?
Also, if "v" is indeed 3.13 m/s calculated from energy conservation as shown in posting #1, then it must be the speed.
In that case, the equation

F= Δp / Δt = (2 x 0.0005 x 3.13 ) / (1/100) = 0.313N

is, strictly speaking, incorrect. If the bead reaches the same height after the collision, its speed must be the same just before and just after the collision and therefore does not change. What changes is the vertical component of the velocity. It would be cleaner to use kinematics to calculate this vertical component just before the collision and label it v_y rather than v in the momentum transfer equations.

 

[hquang001]

Is the "original height" to which the beads bounce back given some place and I missed it?
Also, if "v" is indeed 3.13 m/s calculated from energy conservation as shown in posting #1, then it must be the speed.
In that case, the equation

is, strictly speaking, incorrect. If the bead reaches the same height after the collision, its speed must be the same just before and just after the collision and therefore does not change. What changes is the vertical component of the velocity. It would be cleaner to use kinematics to calculate this vertical component just before the collision and label it v_y rather than v in the momentum transfer equations.

Oh i missed it, the height should be 0.5 m.
and yes the speed does not change that's why the Δp = m( v -(-v) ) but it's not really clear written as you said

 

[jbriggs444]

How can i apply the rate of 100 per second in this equation ?

View attachment 279048

Personally, I would attack this problem from a different angle. Make it about a system. Draw the boundaries of your system at the point where beads enter the system from the left horizontally, at the point where they exit the system on the right horizontally and at the bottom where they interact with the pan.

The number of beads in the system is constant (plus or minus one bead - negligible) and its vertical momentum is constant (on average). So enumerate the places where momentum is gained or lost and write down a momentum balance.

It may be useful to realize that a force and a rate of transfer of momentum over time are essentially the same thing.