- #1
unscientific
- 1,734
- 13
Taken from my lecturer's notes, how did they make the jump from 8.5 to 8.6 and 8.7?
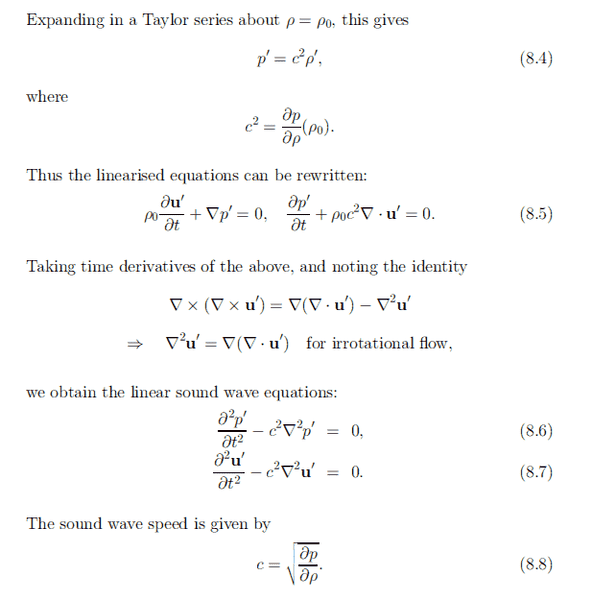
Even after differentiating (8.5) with time I get
[tex] \rho_0 \frac{\partial^2 \vec u'}{\partial t^2} + \nabla \frac{\partial p '}{\partial t} = 0 [/tex]
[tex] \frac{\partial^2 p'}{\partial t^2} + \rho_0 c^2 \nabla \cdot \frac{\partial \vec u'}{\partial t} = 0 [/tex]
Is there a relation between ##\vec u## and ##p## I am missing?
Even after differentiating (8.5) with time I get
[tex] \rho_0 \frac{\partial^2 \vec u'}{\partial t^2} + \nabla \frac{\partial p '}{\partial t} = 0 [/tex]
[tex] \frac{\partial^2 p'}{\partial t^2} + \rho_0 c^2 \nabla \cdot \frac{\partial \vec u'}{\partial t} = 0 [/tex]
Is there a relation between ##\vec u## and ##p## I am missing?