Zuzana
- 12
- 1
Hello :)
I try to fit some parameters of the particle (e.g. energy, direction) be means of log-likelihood minimization.
Input data to likelihood function are pulses amplitudes, while Poisson distribution is used. However, the problem is that Poisson distribution is as follows
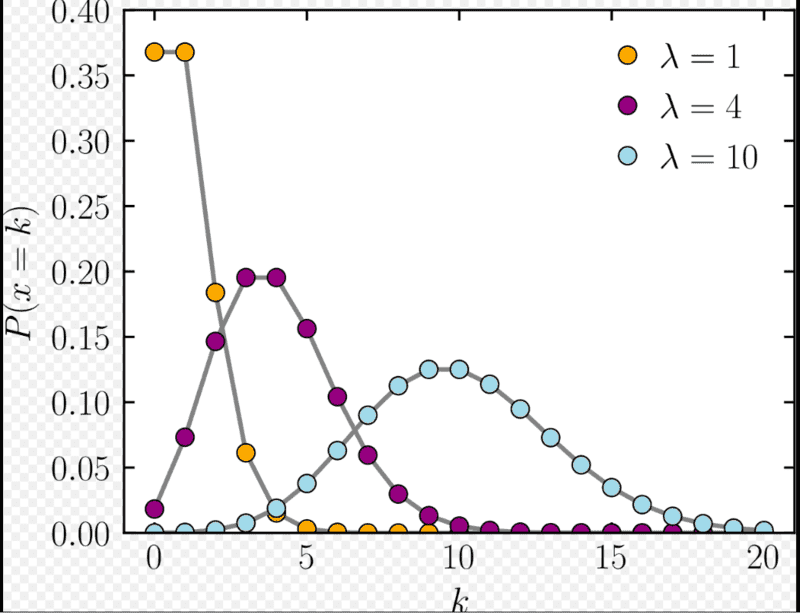
i.e. for higher pulse amplitute there is a lower Poisson probability and thus higher likelihood value. However, pulses with higher amplitudes are very important in the event and the probability for them should be higher, not? Please, do you know how to correct this effect? Or what would you suggest to do?
Thank you very much in advance.
I try to fit some parameters of the particle (e.g. energy, direction) be means of log-likelihood minimization.
Input data to likelihood function are pulses amplitudes, while Poisson distribution is used. However, the problem is that Poisson distribution is as follows
i.e. for higher pulse amplitute there is a lower Poisson probability and thus higher likelihood value. However, pulses with higher amplitudes are very important in the event and the probability for them should be higher, not? Please, do you know how to correct this effect? Or what would you suggest to do?
Thank you very much in advance.