Lorentz Transf. of Complex Null Tetrads: Formula (3.14-17)

[ergospherical]

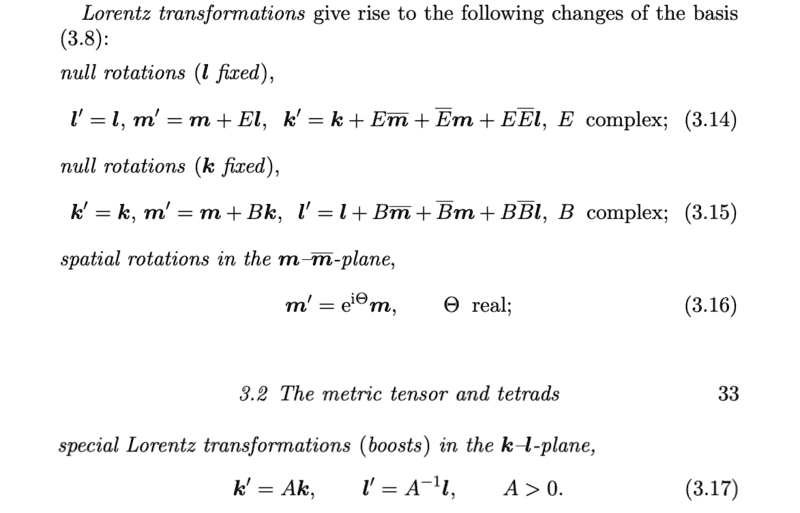
For a complex null tetrad $(\boldsymbol{m}, \overline{\boldsymbol{m}}, \boldsymbol{l}, \boldsymbol{k})$, how to arrive at formulae (3.14), (3.15) and (3.17)? The equation (3.16) is clear as is. (I checked already that they work i.e. that $\boldsymbol{e}_a' \cdot \boldsymbol{e}_b' = 2m'_{(a} \overline{m}'_{b)} -2k'_{(a} l'_{b)}$.)

 

[PeterDonis]

formulae (3.14), (3.15) and (3.17)?

What reference are these from?

 

[George Jones]

What reference are these from?

These are from the second edition of "Exact Solutions of Einstein's Field Equations" by Stephani et al. I have have an elaboration on (3.17), which I have started to type in, but my wife is pulling me away to watch someone get murdered ... er, to stream a show, so it will be a couple of hours before I get back to it.

 

[George Jones]

I have looked at (3.17). I suppose that it is not enough to show that
$$2m'_{(a} \overline{m}'_{b)} -2k'_{(a} l'_{b)} = 2m_{(a} \overline{m}_{b)} -2k_{(a} l_{b)},$$
as this is obvious for transformation (3.17).

I will proceed in a pedestrian way, i.e., I will show that (3.17) defines a boost. Inverting (3.12) gives (using notation that I dislike)
$$\begin{align}
\boldsymbol{E}_4 &= \frac{1}{\sqrt{2}} \left( \boldsymbol{k} +\boldsymbol{l} \right) \\
\boldsymbol{E}_3 &= \frac{1}{\sqrt{2}} \left( \boldsymbol{k} - \boldsymbol{l} \right) .
\end{align}$$
Now define
$$\begin{align}
\boldsymbol{E}'_4 &= \frac{1}{\sqrt{2}} \left( \boldsymbol{k}' +\boldsymbol{l}' \right) \\
\boldsymbol{E}'_3 &= \frac{1}{\sqrt{2}} \left( \boldsymbol{k}' - \boldsymbol{l}' \right) ,
\end{align}$$
with $\boldsymbol{k}'$ and $\boldsymbol{l}'$ given by (3.17). Then, by (3.17),
$$\begin{align}
\boldsymbol{E}'_4 &= \frac{1}{\sqrt{2}} \left( A\boldsymbol{k} +A^{-1} \boldsymbol{l} \right) \\
&= \frac{1}{\sqrt{2}} \left[ \frac{A}{\sqrt{2}} \left( \boldsymbol{E}_4 + \boldsymbol{E_3} \right) + \frac{A^{-1}}{\sqrt{2}} \left( \boldsymbol{E}_4 - \boldsymbol{E_3} \right) \right] \\
&= \frac{1}{2} \left( A + A^{-1} \right) \boldsymbol{E}_4 +\frac{1}{2} \left( A - A^{-1} \right) \boldsymbol{E}_3
\end{align}$$
Since
$$\left[ \frac{1}{2} \left( A + A^{-1} \right) \right]^2 - \left[ \frac{1}{2} \left( A - A^{-1} \right) \right]^2 = 1, $$
we can set
$$\begin{align}
\cosh w &= \frac{1}{2} \left( A + A^{-1} \right) \\
\sinh w &= \frac{1}{2} \left( A - A^{-1} \right)
\end{align}$$
Something similar holds for $\boldsymbol{E}_3$, so we have a boost with rapidity $w$ and speed $v = \tanh w$.