Lorentz Transformations and Angular momentum | Tong's QFT notes

[JD_PM]

TL;DR Summary

I was reading example 1.3.3 of Tong's lecture notes on QFT and I did not understand several points of it.

I have attached the PDF.

I am reading Tong's lecture notes and I found an example in which there are several aspects I do not understand.

This example is aimed at:

- Understanding what is the analogy in field theory to the fact that, in classical mechanics, rotational invariance gives rise to conservation of angular momentum.

Let's start then.

The infinitesimal form of Lorentz transformations is given as

$$\Lambda^{\mu}_{ \ \nu} = \delta^{\mu}_{ \ \nu} + \omega^{\mu}_{ \ \nu}$$

Where $\omega^{\mu}_{ \ \nu}$ is infinitesimal.

We know that the general Lorentz transformation condition is as follows (let's forget about priming indices in this post):

$$\eta^{\mu \nu} = \Lambda^{\mu}_{ \ \sigma} \Lambda^{\nu}_{ \ \tau} \eta^{\sigma \tau}$$

Thus, applying it to the infinitesimal case we get

$$(\delta^{\mu}_{ \ \nu} + \omega^{\mu}_{ \ \nu})(\delta^{\nu}_{ \ \nu} + \omega^{\nu}_{ \ \nu}) \eta^{\sigma \tau} = \eta^{\mu \nu}$$

Let me skip the Algebra; just note that I have dropped the term $\omega^{\mu}_{ \ \sigma} \omega^{\nu \sigma}$. Thus we get

$$\omega^{\mu \nu} = - \omega^{\nu \mu}$$

Thus $\omega^{\mu \nu}$ is antisymmetric. I understand everything at this point.

Then Tong says we can make a check to see that the number of 4x4 antisymmetric matrices is equal to the total number of LTs: 4x3/2=6. This is a basic Linear algebra issue but I do not see it.

Now he shows that the transformation on a scalar field is given by

$$\phi(x) \rightarrow \phi'(x) = \phi(\Lambda^{-1}x) = \phi(x^{\mu} - \omega^{\mu}_{ \ \nu} x^{\nu}) = \phi(x^{\mu}) - \omega^{\mu}_{ \ \nu} x^{\nu} \partial_{\mu} \phi (x)$$

What I do not get is why

$$\phi(\Lambda^{-1}x) = \phi(x^{\mu} - \omega^{\mu}_{ \ \nu} x^{\nu})$$

We know that

$$\Lambda^{-1} = \Lambda^{\nu}_{ \ \mu} = \delta_{ \ \mu}^{\nu} + \omega_{ \ \mu}^{\nu}$$

Thus I get the same but with a positive sign

$$\phi(\Lambda^{-1}x) = \phi(x^{\mu} + \omega^{\mu}_{ \ \nu} x^{\nu})$$

He justifies the sign to be positive when we apply active transformations. Then I think he made a typo and it should be positive (I hqve to say I do not understand why we define two kinds of transformations though: active and positive).

Please let me attach an image of the rest of the example I want to comment:

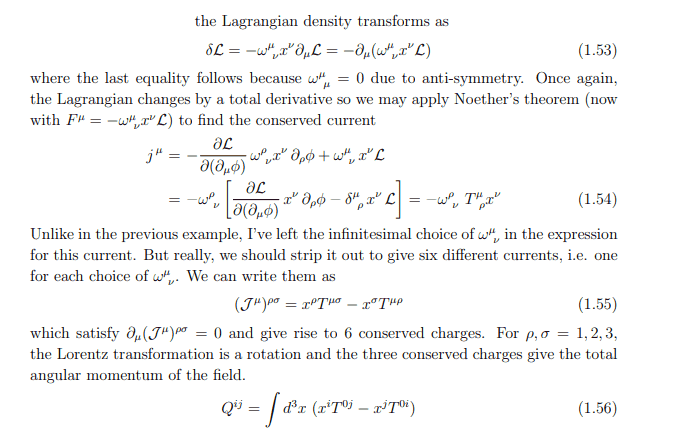
From here I basically do not get the following:

- I do not get the last equality on 1.53 (i.e. why the last equality follows because $\omega^{\mu}_{\mu}=0$; I do not see the antysimmetry argument).

- I do not see neither how to get 1.55 nor $\partial_{\mu}(J^{\mu})^{\rho \sigma} = 0$

I know there are many questions here, so let's summarize:

1) Why The number of 4x4 antisymmetric matrices is equal to the total number of LTs: 4x3/2=6.

2) Why $\phi(\Lambda^{-1}x) = \phi(x^{\mu} - \omega^{\mu}_{ \ \nu} x^{\nu})$

3) How to get equation 1.53

4) How to get equation 1.55

Thank you very much.

 

[PeterDonis]

I have attached the PDF.

Don't attach it; link to it. Where online did you get it from?

 

[vanhees71]

It's here:

https://www.damtp.cam.ac.uk/user/tong/qft.html

Then Tong says we can make a check to see that the number of 4x4 antisymmetric matrices is equal to the total number of LTs: 4x3/2=6. This is a basic Linear algebra issue but I do not see it.

Think about $\omega^{\mu \nu}$ as a $\mathbb{R}^{4 \times 4}$ matrix. If it were unconstrained you'd have 16 independent real numbers in this matrix, but antisymmetry, $\omega^{\mu \nu}=-\omega^{nu \mu}$ are a lot of constraints. First of all the four diagonal elements of the matrix are 0 since antisymmetry means, e.g., $\omega^{11}=-\omega^{11} \; \Rightarrow \; \omega^{11}=0$. This leaves you with the upper triangle of the matrix as the independent entries, i.e., the $\sigma^{\mu \nu}$ with $\mu<\nu$. These are easy to count: $\sigma^{01}$, $\sigma^{02}$, $\sigma^{03}$ (generators of the boosts) and $\sigma^{12}$, $\sigma^{13}$, $\sigma^{23}$ (generators of rotations). The other matrix elements are determined from these 6 numbers due to the antisymmetry of the matrix.

What I do not get is why
$$\phi(\Lambda^{-1}x) = \phi(x^{\mu} - \omega^{\mu}_{ \ \nu} x^{\nu})$$

You only need the inverse of $\Lambda$ to linear order in $\omega$. You know that
$$\eta_{\mu \nu} {\Lambda^{\mu}}_{\rho} {\Lambda^{\nu}}_{\sigma} = \eta_{\rho \sigma}.$$
Contracting with $\eta^{\rho \alpha}$ gives (setting brackets for didactical clarification)
$$(\eta_{\mu \nu} {\Lambda^{\mu}}_{\rho} \eta^{\rho \alpha}) {\Lambda^{\nu}}_{\sigma} = \delta_{\sigma}^{\alpha}$$
or, more conveniently,
$${\Lambda_{\nu}}^{\alpha} {\Lambda^{\nu}}_{\sigma}=\delta_{\sigma}^{\alpha}.$$
On the other hand
$${(\Lambda^{-1})^{\alpha}}_{\nu} {\Lambda^{\nu}}_{\sigma}=\delta_{\sigma}^{\alpha}.$$
Since the inverse of a matrix is unique, this implies
$${(\Lambda^{-1})^{\alpha}}_{\nu}={\Lambda_{\nu}}^{\alpha}$$
and thus for an infinitesimal transformation
$${(\Lambda^{-1})^{\alpha}}_{\nu}=\eta_{\nu \mu} \eta^{\alpha \rho} {\Lambda^{\mu}}_{\rho} = \eta_{\nu \mu} \eta^{\alpha \rho}(\delta_{\rho}^{\mu} + {\omega^{\mu}}_{\rho}) = \delta_{\nu}^{\alpha} + {\omega_{\nu}}^{\alpha}=\delta_{\nu}^{\alpha} + \eta_{\nu \beta} \omega^{\beta \alpha} = \delta_{\nu}^{\alpha} -\eta_{\nu \beta} \omega^{\alpha \beta}=\delta_{\nu}^{\alpha} -{\omega^{\alpha}}_{\nu}.$$
This implies
$$(\Lambda^{-1} x)^{\mu} = x^{\mu} - {\omega^{\mu}}_{\nu} x^{\nu}.$$
From this (in linear order in $\omega$) through Taylor expansion around $x$
$$\phi'(x)=\phi(\Lambda^{-1} x)=\phi(x) - {\omega^{\mu}}_{\nu} x^{\nu} \partial_{\mu} \phi(x) + \mathcal{O}(\omega^2).$$

- I do not get the last equality on 1.53

$$\partial_{\mu} ({\omega^{\mu}}_{\nu} x^{\nu} \mathcal{L}) = {\omega^{\mu}_{\nu}}(\delta_{\mu}^{\nu} \mathcal{L} + x^{\nu} \partial_{\mu} \mathcal{L})={\omega^{\mu}_{\nu}}x^{\nu} \partial_{\mu} \mathcal{L},$$
because due to the antisymmetry of $\omega^{\alpha \beta}$ as well ass the symmetry of $\eta_{\mu \nu}$
$${\omega^{\mu}_{\nu}}\delta_{\mu}^{\nu} =\omega^{\mu \nu} \eta_{\mu \nu} = \frac{1}{2} (\omega^{\mu \nu} - \omega^{\nu \mu}) \eta_{\mu \nu} = \eta_{\mu \nu} \omega^{\mu \nu} - \omega^{\nu \mu} \eta_{\nu \mu} = 0.$$
Noether's theorem tells you that (1.55) is conserved, but you want to get rid of the matrix $\omega$. But the matrix $\omega$ can be arbitrarily chosen under the constraint that $\omega_{\mu \nu}$ is antisymmetric. So we rewrite the current a bit
$$j^{\mu} = -\omega_{\rho \nu} T^{\mu \rho} x^{\nu}=-\frac{1}{2} \omega_{\rho \nu} (T^{\mu \rho} x^{\nu} - T^{\mu \nu} x^{\rho}).$$
Now you can put for $\omega_{\rho \nu}$ all antisymmetric matrices, thus $\partial_{\mu} j^{\mu}=0$ implies that the $(\mathcal{J}^{\mu})^{\rho \sigma}$ are indeed 6 independent conserved currents, $\partial_{\mu} (\mathcal{J}^{\mu})^{\rho \sigma}=0$.

I hope this helps a bit further.

 

[JD_PM]

It's here:

https://www.damtp.cam.ac.uk/user/tong/qft.htmlThink about $\omega^{\mu \nu}$ as a $\mathbb{R}^{4 \times 4}$ matrix. If it were unconstrained you'd have 16 independent real numbers in this matrix, but antisymmetry, $\omega^{\mu \nu}=-\omega^{nu \mu}$ are a lot of constraints. First of all the four diagonal elements of the matrix are 0 since antisymmetry means, e.g., $\omega^{11}=-\omega^{11} \; \Rightarrow \; \omega^{11}=0$. This leaves you with the upper triangle of the matrix as the independent entries, i.e., the $\sigma^{\mu \nu}$ with $\mu<\nu$. These are easy to count: $\sigma^{01}$, $\sigma^{02}$, $\sigma^{03}$ (generators of the boosts) and $\sigma^{12}$, $\sigma^{13}$, $\sigma^{23}$ (generators of rotations). The other matrix elements are determined from these 6 numbers due to the antisymmetry of the matrix.You only need the inverse of $\Lambda$ to linear order in $\omega$. You know that
$$\eta_{\mu \nu} {\Lambda^{\mu}}_{\rho} {\Lambda^{\nu}}_{\sigma} = \eta_{\rho \sigma}.$$
Contracting with $\eta^{\rho \alpha}$ gives (setting brackets for didactical clarification)
$$(\eta_{\mu \nu} {\Lambda^{\mu}}_{\rho} \eta^{\rho \alpha}) {\Lambda^{\nu}}_{\sigma} = \delta_{\sigma}^{\alpha}$$
or, more conveniently,
$${\Lambda_{\nu}}^{\alpha} {\Lambda^{\nu}}_{\sigma}=\delta_{\sigma}^{\alpha}.$$
On the other hand
$${(\Lambda^{-1})^{\alpha}}_{\nu} {\Lambda^{\nu}}_{\sigma}=\delta_{\sigma}^{\alpha}.$$
Since the inverse of a matrix is unique, this implies
$${(\Lambda^{-1})^{\alpha}}_{\nu}={\Lambda_{\nu}}^{\alpha}$$
and thus for an infinitesimal transformation
$${(\Lambda^{-1})^{\alpha}}_{\nu}=\eta_{\nu \mu} \eta^{\alpha \rho} {\Lambda^{\mu}}_{\rho} = \eta_{\nu \mu} \eta^{\alpha \rho}(\delta_{\rho}^{\mu} + {\omega^{\mu}}_{\rho}) = \delta_{\nu}^{\alpha} + {\omega_{\nu}}^{\alpha}=\delta_{\nu}^{\alpha} + \eta_{\nu \beta} \omega^{\beta \alpha} = \delta_{\nu}^{\alpha} -\eta_{\nu \beta} \omega^{\alpha \beta}=\delta_{\nu}^{\alpha} -{\omega^{\alpha}}_{\nu}.$$
This implies
$$(\Lambda^{-1} x)^{\mu} = x^{\mu} - {\omega^{\mu}}_{\nu} x^{\nu}.$$
From this (in linear order in $\omega$) through Taylor expansion around $x$
$$\phi'(x)=\phi(\Lambda^{-1} x)=\phi(x) - {\omega^{\mu}}_{\nu} x^{\nu} \partial_{\mu} \phi(x) + \mathcal{O}(\omega^2).$$

$$\partial_{\mu} ({\omega^{\mu}}_{\nu} x^{\nu} \mathcal{L}) = {\omega^{\mu}_{\nu}}(\delta_{\mu}^{\nu} \mathcal{L} + x^{\nu} \partial_{\mu} \mathcal{L})={\omega^{\mu}_{\nu}}x^{\nu} \partial_{\mu} \mathcal{L},$$
because due to the antisymmetry of $\omega^{\alpha \beta}$ as well ass the symmetry of $\eta_{\mu \nu}$
$${\omega^{\mu}_{\nu}}\delta_{\mu}^{\nu} =\omega^{\mu \nu} \eta_{\mu \nu} = \frac{1}{2} (\omega^{\mu \nu} - \omega^{\nu \mu}) \eta_{\mu \nu} = \eta_{\mu \nu} \omega^{\mu \nu} - \omega^{\nu \mu} \eta_{\nu \mu} = 0.$$
Noether's theorem tells you that (1.55) is conserved, but you want to get rid of the matrix $\omega$. But the matrix $\omega$ can be arbitrarily chosen under the constraint that $\omega_{\mu \nu}$ is antisymmetric. So we rewrite the current a bit
$$j^{\mu} = -\omega_{\rho \nu} T^{\mu \rho} x^{\nu}=-\frac{1}{2} \omega_{\rho \nu} (T^{\mu \rho} x^{\nu} - T^{\mu \nu} x^{\rho}).$$
Now you can put for $\omega_{\rho \nu}$ all antisymmetric matrices, thus $\partial_{\mu} j^{\mu}=0$ implies that the $(\mathcal{J}^{\mu})^{\rho \sigma}$ are indeed 6 independent conserved currents, $\partial_{\mu} (\mathcal{J}^{\mu})^{\rho \sigma}=0$.

I hope this helps a bit further.

I will read it carefully, thank you.

 

[JD_PM]

Vanhees71 thank you very much, your explanation made me understand almost everything.

Here's my issue now: I do not see why the following holds

$$\partial_{\mu} ({\omega^{\mu}}_{\nu} x^{\nu} \mathcal{L}) = {\omega^{\mu}_{\nu}}(\delta_{\mu}^{\nu} \mathcal{L} + x^{\nu} \partial_{\mu} \mathcal{L})$$

I would expect instead to have the following

$$\partial_{\mu} ({\omega^{\mu}}_{\nu} x^{\nu} \mathcal{L}) = \omega^{\mu}_{\nu} x^{\nu} \partial_{\mu} \mathcal{L}$$

Thus, what I do not see is why the term $\omega^{\mu}_{\nu}\delta_{\mu}^{\nu} \mathcal{L}$ arises.

 

[vanhees71]

It's from the product rule and
$$\partial_{\mu} x^{\nu} = \delta_{\mu}^{\nu}.$$

 

[JD_PM]

It's from the product rule and
$$\partial_{\mu} x^{\nu} = \delta_{\mu}^{\nu}.$$

Ahh sorry Sr, I see it now! Thank you again.

I forgot that

$$\partial_{\mu} x^{\nu} = \frac{\partial x^{\nu}}{\partial x^{\mu}} = \delta_{\mu}^{\nu}.$$