Lorentz transformations for electric and magnetic fields

[Frostman]

Homework Statement

In an inertial reference system $S$ there are an electric field $\overline{E}$ and a magnetic field $\overline{B}$, uniform and constant, which form an angle $\theta$ with $0<\theta<\frac \pi 2$ between them.
1 - What completely general conclusions can be drawn about the fields $\overline{E'}$ and $\overline{B'}$, seen in a generic inertial frame of reference $S'$?
2- Calculate, if possibile, the speed $\overline{v}$ of the reference system $S'$ in which the fields are perpendicular. Justify the answer.
3- Calculate, if possible, the speed $\overline{v}$ of the inertial reference system in which the fields are parallel. Justify the answer.

Relevant Equations

Lorentz transformations

Good evening, I'm trying to solve this exercise that apparently seems trivial, but I wouldn't want to make mistakes, just trivial. Proceeding with the first point I wonder if my approach can be correct in describing this situation.

1 - What completely general conclusions can be drawn about the fields $\overline{E'}$ and $\overline{B'}$, seen in a generic inertial frame of reference $S'$?

From the assumptions, the two fields are in this configuration:

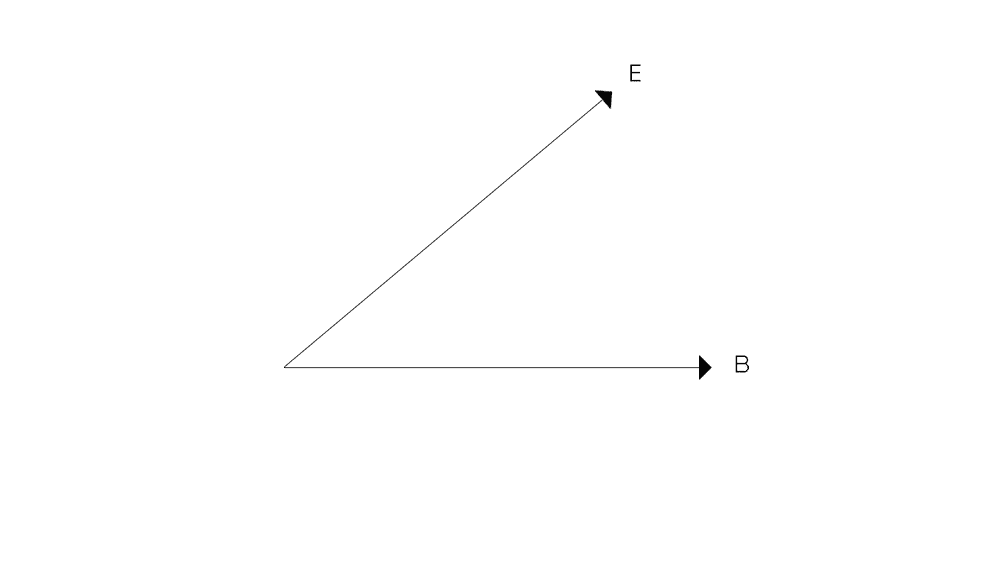I can observe that if the motion occurs along one of the two directions of the fields, in passing from one SRI to another, there will be a different value of the orthogonal component which it takes place in motion. If the motion occurs along the direction of the electric field, it will remain sent as it passes from one SRI to another. While the magnetic field will modify its orthogonal component to the electric field. In a similar way the vice versa holds true. In general, therefore, we can say that the components of the electric and magnetic fields parallel to the direction of motion will not undergo any change when passing from one SRI to another, while the orthogonal components will change. The inverse of what happened for the four-position and four-pulse occurs.

 

[anuttarasammyak]

If you are familiar with electro magnetic tensor F,
$$F_{\mu\nu}F^{\mu\nu}=invariant$$
$$e^{\mu\nu\eta\zeta}F_{\mu\nu}F_{\eta\zeta}=invariant$$
give two invariant quantity under Lorentz transformation.

 

[Frostman]

Okkay, I can find these Lorentz invariant

$$F_{\mu\nu}F^{\mu\nu}=invariant$$

$$
F^{\mu\nu}F_{\mu\nu}=F^{0i}F_{0i}+F^{i0}F_{i0}+F^{ij}F_{ij}=-|E|^2-|E|^2+2|B|^2=-2\big(|E|^2-|B|^2\big)
$$

$$e^{\mu\nu\eta\zeta}F_{\mu\nu}F_{\eta\zeta}=invariant$$

$$
F^{*\alpha\beta}F_{\alpha\beta}=\frac 12 \varepsilon^{\alpha\beta\gamma\delta}F_{\gamma\delta}F_{\alpha\beta}=F^{*0i}F_{i0}+F^{*i0}F_{i0}+F^{*ij}F_{ij}=-B_xE_x-B_yE_y-B_zE_z-E\cdot B - 2E \cdot B = -4 E\cdot B
$$

 

[anuttarasammyak]

The system has constant Poynting vector oriented to z direction which we cannot cancel by Lorentz transformation. That suggests both E and B are not zero nor E $\parallel$ B in any IFR. Your results back it.

EDIT
False: cannot cancel, nor $E \parallel B$
Correct: can cancel, could be $E\parallel B$

 

[Frostman]

The system has constant Poynting vector oriented to z direction which we cannot cancel by Lorentz transformation. That suggests both E and B are not zero nor E $\parallel$ B in any IFR. Your results back it.

I'm not following you...
The Poynting vector is defined as $S = E \times B$, while with the electromagnetic tensor $F^{\mu\nu}$ we have proved two different quantities to be Lorentz-invariant.

Of these two quantities ($E^2-B^2$ e $E\cdot B$) I can say that:

1. When we have orthogonal fields ($E \perp B$) then $E\cdot B = 0$, so there is an IFR where we have $E = 0$ or $B = 0$.
2. Situation in which $E = 0$ or $B = 0$: it must necessarily be that in every IFR $E \cdot B = 0$, that is, we have $E \perp B$
3. If you have an IRF where $|E| = |B|$ then $|E|^ 2-|B|^2 = 0$ in each IRF, so the modulus of the two fields is the same in each IRF.

 

[anuttarasammyak]

I would rather say

If $E^2-B^2=inv<0$ then $B \neq 0$ in any IFR. No cancellation of magnetic field, like field generated by magnet.
If $E^2-B^2=inv=0$ then $E^2=B^2$ in any IFR, like electro magnetic wave.
If $E^2-B^2=inv>0$ then $E \neq 0$ in any IFR. No cancellation of electric field, like field generated by charge.

If $E\cdot B =inv=0$ then $E\cdot B=0$ in any IFR so the two fields are perpendicular, like electro magnetic wave.
If $E\cdot B =inv\neq 0$ then $E\cdot B\neq0$ in any IFR so $E\neq 0$ and $B\neq 0$ in any IFR so there would be a IFR where the two fields are parallel. This is the case of the problem.

 

[Frostman]

If $E\cdot B =inv=0$ then $E\cdot B=0$ in any IFR so the two fields are perpendicular, like electro magnetic wave.

But I can't have this situation because $\theta \in (0, \frac \pi2)$. So for the request:

2- Calculate, if possibile, the speed $\overline v$ of the reference system $S′$ in which the fields are perpendicular. Justify the answer.

They could be perpendicular, but for hypothesis $\theta \in (0, \frac \pi2)$

If $E\cdot B =inv\neq 0$ then $E\cdot B\neq0$ in any IFR so $E\neq 0$ and $B\neq 0$ in any IFR so there would be a IFR where the two fields are parallel. This is the case of the problem.

Then there is an infinity of reference systems that satisfy the condition. Therefore, having found one, every other system that moves relative to the first with direct velocity along the common direction of the fields enjoys the same property. It will therefore be sufficient to determine between these systems a system whose speed is perpendicular to both fields. Choosing the x-axis for the direction of velocity and using the fact that in the system $S'$: $E_x' = B_x'= 0$, $E_y'B_y'-E_z'B_z' = 0$ using the formulas of passage between IRF for the speed $v$ of the system $S'$ with respect to the initial system I get:
$$
\frac{v}{1-v^2}=\frac{E\cdot B}{E^2 +B^2}
$$
In this case I can find a $v$ (remembering that $v<1$ in NU). I satisfied the third point:

3- Calculate, if possible, the speed $\overline v$ of the inertial reference system in which the fields are parallel. Justify the answer.

Let me know if is all right.

 

[anuttarasammyak]

You may check your assumption directly by formula of Lorentz transformation, i.e.

$$E_x=\gamma(E_x'+vB_y')$$
$$E_y=\gamma(E_y'-vB_x')$$
$$B_x=\gamma(B_x'-vE_y')$$
$$B_y=\gamma(B_y'+vE_x')$$
$$E_z=E_z'=0$$
$$B_z=B_z'=0$$
where v has direction z.

Ref. my comment #4 the IFR where $E\parallel B$ so there is no Poynting vector is CoM system of EM field energy and momentum.