- 1,097
- 1,384
Need help with part iii)
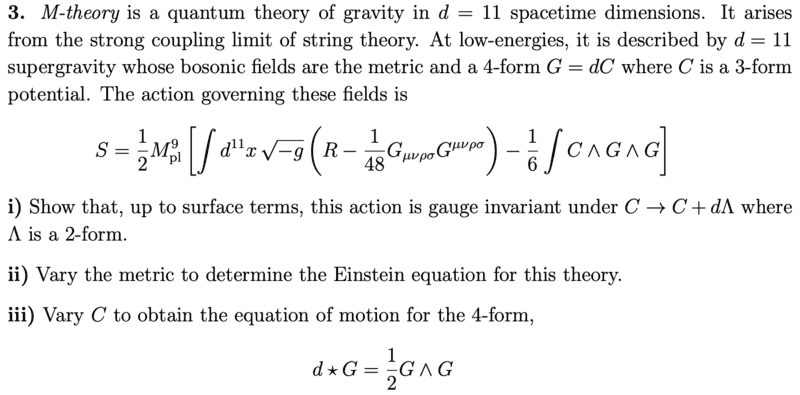
i) Under ##C \rightarrow C + d\Lambda##, and since ##dG = d^2C = 0 \implies d(\Lambda \wedge G \wedge G) = d\Lambda \wedge G \wedge G##, then neglecting the surface terms\begin{align*}
\int_D d\Lambda \wedge G \wedge G = \int_D d(\Lambda \wedge G \wedge G) &= \int_{\partial D} \Lambda \wedge G \wedge G = 0
\end{align*}ii) Varying with respect to the metric\begin{align*}
\delta S = \dfrac{1}{2}M^9 \int d^{11}x \left[\delta\sqrt{-g} \left(R - \dfrac{1}{48}G_{\mu \nu \rho \sigma}G^{\mu \nu \rho \sigma} \right) + \sqrt{-g} \delta R \right]
\end{align*}Using the classic trick for diagonalisable matrices ##\delta \sqrt{-g} = \dfrac{1}{2\sqrt{-g}} (-g) \mathrm{tr}(g^{-1} \delta g) = \dfrac{-1}{2} \sqrt{-g} g_{\mu \nu} \delta g^{\mu \nu}##. Meanwhile for the Ricci scalar\begin{align*}
\delta R = \delta (g^{\mu \nu} R_{\mu \nu}) &= g^{\mu \nu} \delta R_{\mu \nu} + \delta g^{\mu \nu}R_{\mu \nu} \\
&= \nabla_{\mu} [g^{\rho \nu} \delta \Gamma^{\mu}_{\rho \nu} - g^{\mu \nu} \delta \Gamma^{\rho}_{\nu \rho}] + \delta g^{\mu \nu} R_{\mu \nu}
\end{align*}Therefore the Einstein equation should be\begin{align*}
\dfrac{-1}{2} g_{\alpha \beta} \left(R - \dfrac{1}{48}G_{\mu \nu \rho \sigma}G^{\mu \nu \rho \sigma} \right) + R_{\alpha \beta} &= 0 \\
\end{align*}Is this correct?
iii) Little progress made, hints appreciated.
i) Under ##C \rightarrow C + d\Lambda##, and since ##dG = d^2C = 0 \implies d(\Lambda \wedge G \wedge G) = d\Lambda \wedge G \wedge G##, then neglecting the surface terms\begin{align*}
\int_D d\Lambda \wedge G \wedge G = \int_D d(\Lambda \wedge G \wedge G) &= \int_{\partial D} \Lambda \wedge G \wedge G = 0
\end{align*}ii) Varying with respect to the metric\begin{align*}
\delta S = \dfrac{1}{2}M^9 \int d^{11}x \left[\delta\sqrt{-g} \left(R - \dfrac{1}{48}G_{\mu \nu \rho \sigma}G^{\mu \nu \rho \sigma} \right) + \sqrt{-g} \delta R \right]
\end{align*}Using the classic trick for diagonalisable matrices ##\delta \sqrt{-g} = \dfrac{1}{2\sqrt{-g}} (-g) \mathrm{tr}(g^{-1} \delta g) = \dfrac{-1}{2} \sqrt{-g} g_{\mu \nu} \delta g^{\mu \nu}##. Meanwhile for the Ricci scalar\begin{align*}
\delta R = \delta (g^{\mu \nu} R_{\mu \nu}) &= g^{\mu \nu} \delta R_{\mu \nu} + \delta g^{\mu \nu}R_{\mu \nu} \\
&= \nabla_{\mu} [g^{\rho \nu} \delta \Gamma^{\mu}_{\rho \nu} - g^{\mu \nu} \delta \Gamma^{\rho}_{\nu \rho}] + \delta g^{\mu \nu} R_{\mu \nu}
\end{align*}Therefore the Einstein equation should be\begin{align*}
\dfrac{-1}{2} g_{\alpha \beta} \left(R - \dfrac{1}{48}G_{\mu \nu \rho \sigma}G^{\mu \nu \rho \sigma} \right) + R_{\alpha \beta} &= 0 \\
\end{align*}Is this correct?
iii) Little progress made, hints appreciated.
Last edited: