- #1
XXZZ
- 6
- 0
Homework Statement
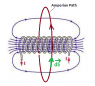
I found solution to problem 257 from "300 Creative Physics problems" hard to understand. In that problem we have very long solenoid with coil wounded in one layer. Data such as: density of turns, magnetic field at distance of 5 cm form the axis of solenoid and diameter (1 cm) was given. We hevo to calculate field inside the solenoid. Solution states, that "Because of the symmetry of arrangement, the magnetic field produced by the coil outside the solenoid is similar to that of a straight, current-carrying wire, as it is shown in the figure on page 474 [picture attached]."
Homework Equations
The Attempt at a Solution
Then, author used that fact and applied Maxwell second law to circle of radius 5cm. IMO, quoted text is meaningless. Magnetic field lines, which are eascaping interior of solenoid must "return" and re-enter that (Gauss law for magnetism). Also, they will never be perpendicular to the lines inside the solenoid, so they cannot be treated as circular field (produced by straight conducting wire). Please help!