Magnetic Field [Perpendicular wires]

[Antonius]

Homework Statement

Homework Equations

Biot-Savart Law: ## dB = μIdl/4πr^2
F = ILxB

The Attempt at a Solution

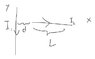
I have not tried to solve it. BUT, please check my approach. I want to make sure my method is correct and whether there is a flaw or no.

I am trying to set up an integral here. I am to integrate it from from $d+L$ to $d$. To do so, I will ignore the current in $I_2$ while integrating. Then, once I found $B$ created by $I_1$ (which is "into the plane." [Meaning force acting on $I_2$ is in the same direction as $I_2$

What is wrong up until now?

Well, here I am not sure how to find F acting on $I_2$... Does $F = IL(dot)B$ work?

Thank you.

 

[Mister T]

I don't think you need to integrate along the infinite wire to find the magnetic field $\vec{B}$ it creates. You can just use the well-known result.

But you will need to integrate along the short wire, using the limits of integration you mentioned. Integrate

$d \vec{F}=I \ d \vec{L} \times \vec {B}$

to find the force.

 

[Antonius]

I don't think you need to integrate along the infinite wire to find the magnetic field $\vec{B}$ it creates. You can just use the well-known result.

But you will need to integrate along the short wire, using the limits of integration you mentioned. Integrate

$d \vec{F}=I \ d \vec{L} \times \vec {B}$

to find the force.

So, I can just use B = μI/2πr, where r is distance from wire 1 to wire 2, to find the Magnetic field that $I_1$ creating?

Can you shortly explain the reason behind not integrating to find $B$? I am not quite clear on that...

 

[Mister T]

So, I can just use B = μI/2πr, where r is distance from wire 1 to wire 2, to find the Magnetic field that $I_1$ creating?

The distance from (the closest point along) Wire 1 to each differential element $d \vec{L}$ in Wire 2, yes.

Can you shortly explain the reason behind not integrating to find $B$? I am not quite clear on that...

Integrating along the infinitely-long Wire 1 to determine the magnetic field $\vec {B}$ will result in the well-known expression $\frac{\mu_oI}{2 \pi r}$ for its magnitude. You will then have to use that result to perform the other integration (the one I described in my first post) to find $\vec{F}$. I don't think it's your prof's intention that you do both integrals as the first one is found in every textbook.