- #1
- 11
- 1
- Homework Statement
- In the circuit shown in picture, having resistance R, a bar of mass m and lenght l moves without friction with velocity v= l/T. The circuit is at time t=0 in a regione with no magnetic field, then in the first part (-l/2<= x <0) the magnetic field is constant and equal to -B0, later it changes to +B0 (0< x <=l/2). We are considering what happens during 0<t<=T. Find current flowing in the circuit, force that needs to be applied to keep the bar moving at constant speed and (something else I don't know how to traslate in english: power balance maybe?)
- Relevant Equations
- Faraday's law, others
Sorry if I post again about this topic (last time I promise!) but I still have some doubts regarding the concept of flux. This collection of problems I have quite standard but there are so many variations. Here is the circuit in question:
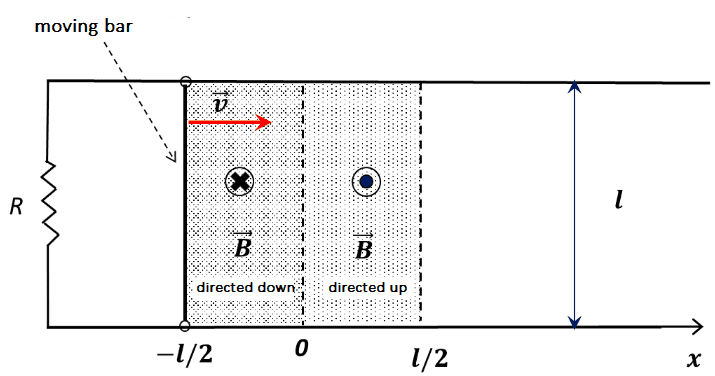
Something tells me that I could write a function that spans the whole region and then integrate... Maybe I could use ##B(x) = |B_{0}|##, but doesn't look convenient... So I just do as if those two regions were independent.
Region ##-\frac{l}{2} \leq x<0:## the bar moves to the right while the magnetic force opposes the movement. Not sure if it's going to get in the second region, but I guess so otherwise the problem would not be like this
. The flux is : ##\Phi = -B_{0}lx(t)##, the current is computed with Faraday's law and it flows in clock direction.
##x = 0:## Here the flux should be discontinous... Does it really matter?
Region ##0 \leq x<\frac{l}{2}:## supposing the bar comes in this region with a positive velocity, then the current should flow in anticlock direction. Given the fact that the initial velocity is given as ##\frac{l}{T}## and the whole thing happens in a time between 0 and T, I think that at some point the bar will stop. But these were just my thoughts, this problem looks a bit long to solve but not impossible, I am going to solve it afterwards...
##\textbf{My doubt actually is:}## if I change the ##x = 0## and move it to ##-l/2##, can I write the flux as only one function? I mean ##\Phi = B_{0}lx(t)## with ##0<=x<l/2, l/2 < x <= l## or is it more complex than that and I am missing something?
Something tells me that I could write a function that spans the whole region and then integrate... Maybe I could use ##B(x) = |B_{0}|##, but doesn't look convenient... So I just do as if those two regions were independent.
Region ##-\frac{l}{2} \leq x<0:## the bar moves to the right while the magnetic force opposes the movement. Not sure if it's going to get in the second region, but I guess so otherwise the problem would not be like this
. The flux is : ##\Phi = -B_{0}lx(t)##, the current is computed with Faraday's law and it flows in clock direction.##x = 0:## Here the flux should be discontinous... Does it really matter?
Region ##0 \leq x<\frac{l}{2}:## supposing the bar comes in this region with a positive velocity, then the current should flow in anticlock direction. Given the fact that the initial velocity is given as ##\frac{l}{T}## and the whole thing happens in a time between 0 and T, I think that at some point the bar will stop. But these were just my thoughts, this problem looks a bit long to solve but not impossible, I am going to solve it afterwards...
##\textbf{My doubt actually is:}## if I change the ##x = 0## and move it to ##-l/2##, can I write the flux as only one function? I mean ##\Phi = B_{0}lx(t)## with ##0<=x<l/2, l/2 < x <= l## or is it more complex than that and I am missing something?