Magnetic Potential & Magnetic Field

[MMS]

Homework Statement
I'm given an infinite cylinder with radius a. There's a uniformal current density j flowing in the cylinder in the z direction.
I'm asked to find the magnetic field in the following ways
-Ampere's law
-Magnetic potential and then use it to calculate the magnetic field.

The attempt at a solution
Calculating the magnetic field by Ampere's law wasn't a problem.

I got stuck on the second part a little. The attempt is in the file below.

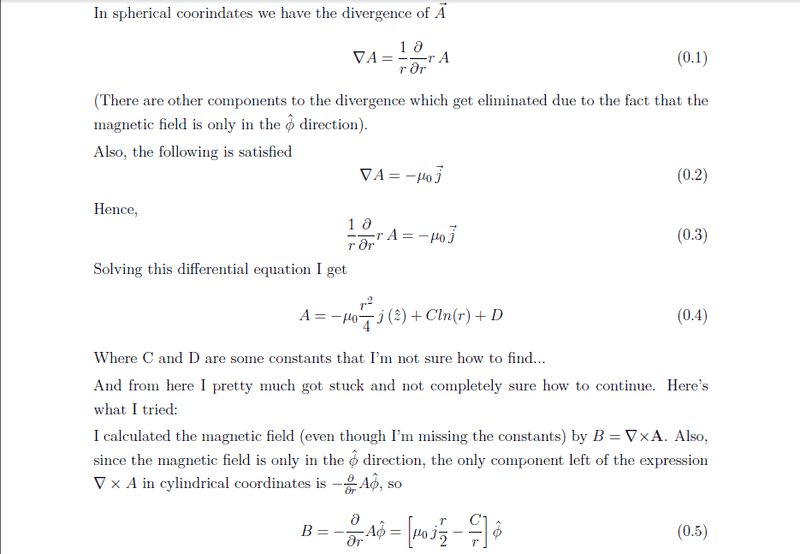
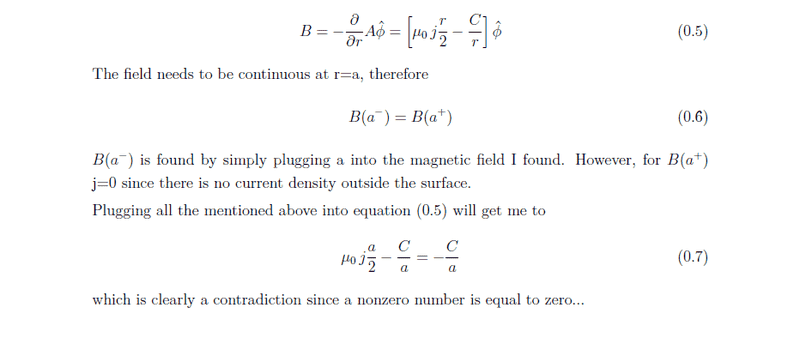
Help ASAP will be very much appreciated since I have to hand this homework tomorrow.

Thank you!

 

[Orodruin]

First of all, the divergence of A is a scalar and you are equating it with the current density, which is a vector. Already here you should start suspecting something is wrong. The divergence you quote is also only valid for a purely radial field, not a field in the phi direction.

Help ASAP will be very much appreciated since I have to hand this homework tomorrow.

This really does nothing but to make you appear impatient and only here to complete your homework rather than actually learning something. You will find that our members (who are here to help on a pro bono basis) are usually reluctant to provide help in these cases.

 

[MMS]

First of all, the divergence of A is a scalar and you are equating it with the current density, which is a vector. Already here you should start suspecting something is wrong. The divergence you quote is also only valid for a purely radial field, not a field in the phi direction.

This really does nothing but to make you appear impatient and only here to complete your homework rather than actually learning something. You will find that our members (who are here to help on a pro bono basis) are usually reluctant to provide help in these cases.

Apologies. I was pretty frustrated with this question back then as I spent a couple of good hours trying to get to something.
I finally derived some things and I believe I solved it taking your notes into consideration. :)