- #1
crisicola
- 2
- 0
Hi, this my first time asking a question, so here it goes:
A 255 kg piano slides 4.6 m down a 30° incline and is kept from accelerating by a man who is pushing back on it parallel to the incline (Fig. 6-35). The effective coefficient of kinetic friction is 0.40.
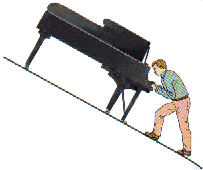
(a) Calculate the force exerted by the man.
So far, I have this answer, by subtracting the Fparallel(mg*sin(30)) by Ffriction(mu*mg*cos(30)). [383.8 N]
(b) Calculate the work done by the man on the piano.
Got this answer, too, by multiplying the answer above by 4.6m. [-1764.6 J]
It's the next parts I got wrong.
(c) Calculate the work done by the friction force.
I figured Ffriction = mu * m * g * cos(30) = 865.7 * d = 3982.12 J, but that doesn't make sense. [?J]
(d) What is the work done by the force of gravity?
9.8 m/s^2 * 255g * 4.6m = 11495.4, it's incorrect [?J]
(e) What is the net work done on the piano?
This one I had no idea how to do altogether. J
Please help me out, thank you in advance!
A 255 kg piano slides 4.6 m down a 30° incline and is kept from accelerating by a man who is pushing back on it parallel to the incline (Fig. 6-35). The effective coefficient of kinetic friction is 0.40.
(a) Calculate the force exerted by the man.
So far, I have this answer, by subtracting the Fparallel(mg*sin(30)) by Ffriction(mu*mg*cos(30)). [383.8 N]
(b) Calculate the work done by the man on the piano.
Got this answer, too, by multiplying the answer above by 4.6m. [-1764.6 J]
It's the next parts I got wrong.
(c) Calculate the work done by the friction force.
I figured Ffriction = mu * m * g * cos(30) = 865.7 * d = 3982.12 J, but that doesn't make sense. [?J]
(d) What is the work done by the force of gravity?
9.8 m/s^2 * 255g * 4.6m = 11495.4, it's incorrect [?J]
(e) What is the net work done on the piano?
This one I had no idea how to do altogether. J
Please help me out, thank you in advance!