Mastering Moments | Cathy's Question Answered

[CathyLou]

Please could someone tell me how to work out the attached moments question as I've really no idea how to do this one.

I'd be really grateful for any help.

Thank you.

Cathy

 

[Hootenanny]

Unfortunately there are not normally many mentors around at this time to approve your attachment, perhaps you could host your image on an external site, such as ImageShack?

 

[CathyLou]

Thanks for your suggestion.

Would it be okay though if I just sent the attatchment to you by a private message instead? It's just that I'm trying to not sign up to any more sites as I receive so much junk mail from sites that I used once or twice years ago.

Cathy

 

[Hootenanny]

Thanks for your suggestion.

Would it be okay though if I just sent the attatchment to you by a private message instead? It's just that I'm trying to not sign up to any more sites as I receive so much junk mail from sites that I used once or twice years ago.

Cathy

I believe that PM attachments also need mentor approval. However, I do know that ImageShack does not require you to sign up or any contact information. You can wait for a mentor to approve your attachment (which usually happens quickly when they're online), I just thought that you would get your question answered more rapidly this way.

 

[CathyLou]

Okay. Thanks for replying. I'll take a look at ImageShack.

Cathy

 

[CathyLou]

Ooh, that was quick!

Here's the link: http://img291.imageshack.us/img291/8981/question10fg0.th.gif

Thanks for the tip!

Cathy

 

[Hootenanny]

No problem. I am assuming that the question requires an numerical answer. If this is the case, first you must find the force exerted at x. For this question one must assume that the seesaw is pivoted about its centre and the seesaw is in equilibrium.

 

[CathyLou]

No problem. I am assuming that the question requires an numerical answer. If this is the case, first you must find the force exerted at x. For this question one must assume that the seesaw is pivoted about its centre and the seesaw is in equilibrium.

Right, so the force at X is equal to 18N (anticlockwise moment = clockwise moment), but what do I do next to find the total downward force on the pivot, and then the reaction force? Would the total downward force be 7.2N + 18N?

Cathy

 

[Astronuc]

Would the total downward force be 7.2N + 18N?

One would sum the applied downward forces AND the weight of the seesaw. If the pivot was off-centre, then one would have to determine the moments of the arms of the see-saw.

 

[CathyLou]

One would sum the applied downward forces AND the weight of the seesaw. If the pivot was off-centre, then one would have to determine the moments of the arms of the see-saw.

Okay, thanks for your help.

Would I use trigonometry next to calculate the value of the reaction force of the pivot up on the seesaw?

Cathy

 

[Hootenanny]

Okay, thanks for your help.

Would I use trigonometry next to calculate the value of the reaction force of the pivot up on the seesaw?

Cathy

Trigonometry is not necessary sum the forces as Astronuc says and then use the fact the the whole system is in equilibrium, in other words the upwards forces must be equal to the downwards forces.

 

[CathyLou]

Trigonometry is not necessary sum the forces as Astronuc says and then use the fact the the whole system is in equilibrium, in other words the upwards forces must be equal to the downwards forces.

Oh, I get it now.

Thanks so much!

Cathy

 

[Hootenanny]

Oh, I get it now.

Thanks so much!

Cathy

Twas a pleasure.

 

[CathyLou]

I'd be really grateful if someone could please give some hints on how to do the next two questions as well.

Here's the link: http://img71.imageshack.us/img71/1157/questions14and15ge1.th.gif

Thank you.

Cathy

 

[Hootenanny]

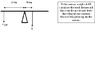
Again, here you need to assume that the bars are in equilibrium (and are uniform), i.e. there is no net torque. The anti clockwise moments must equal the clockwise moments. Note that the weight of the bar will always act through it's centre of mass.

 

[CathyLou]

Again, here you need to assume that the bars are in equilibrium (and are uniform), i.e. there is no net torque. The anti clockwise moments must equal the clockwise moments. Note that the weight of the bar will always act through it's centre of mass.

Sorry to be a pain, but I still really don't get how to find the weight of the bar. Could you please give me an equation and then I'll put in the numbers from the question, as I've no idea what to do. I've looked over all my notes from class and I can't see anything that could help me with these questions.

Would the clockwise moment on the 1st question be 2.7 x 650 (1755N m)?

Thanks.

Cathy

 

[rsk]

Yes, and the moment of the weight is W x its distance from the pivot, which you know (centre of mass is the centre of a uniform beam, ie 1.5m from the end).

Equate the two moments, and you should get a value for W.

 

[CathyLou]

Yes, and the moment of the weight is W x its distance from the pivot, which you know (centre of mass is the centre of a uniform beam, ie 1.5m from the end).

Equate the two moments, and you should get a value for W.

Thank you sooo much!

It was the centre of mass bit that I didn't realize. I get it now though - YAY!

Cathy