Mastering Vector Direction: How to Solve for Unknown Forces in Your Equations

  • Thread starter Thread starter Ry122
  • Start date Start date
  • Tags Tags
    Direction Force
Click For Summary

Discussion Overview

The discussion revolves around determining the direction of vectors in equations related to forces, particularly in the context of static equilibrium and free body diagrams. Participants explore the implications of assumptions made about vector directions and how these affect the formulation of equations.

Discussion Character

  • Exploratory
  • Debate/contested
  • Technical explanation

Main Points Raised

  • Some participants suggest that the direction of vectors A, B, and C may be unknowable at the outset, leading to uncertainty in formulating equations.
  • There is a proposal that if certain vectors are known to point in specific directions (e.g., C opposite to P_x), one could simplify equations by ignoring signs, although the correctness of this approach is questioned.
  • One participant expresses difficulty in understanding the diagram and the directions of vectors A and B, indicating a need for clarity.
  • Another participant emphasizes that assumptions about vector directions are necessary to begin solving the problem, but questions how one would know if those assumptions were incorrect.
  • A different viewpoint suggests that if a free body diagram is used, assumptions may not be needed, as known forces can be projected onto axes to satisfy static equilibrium conditions.
  • One participant humorously suggests that making a guess about vector direction is acceptable, noting that a negative result would indicate an incorrect assumption.

Areas of Agreement / Disagreement

Participants express differing views on whether the direction of vectors can be known or assumed at the outset. There is no consensus on the best approach to determining vector directions or the implications of incorrect assumptions.

Contextual Notes

Some limitations include the vague wording of initial posts, which may lead to misunderstandings about the problem context and the specific forces involved. The discussion also highlights the dependency on clear definitions and assumptions in vector analysis.

Ry122
Messages
563
Reaction score
2
members are reminded that their problem statement and diagrams must be clear and understandable by others
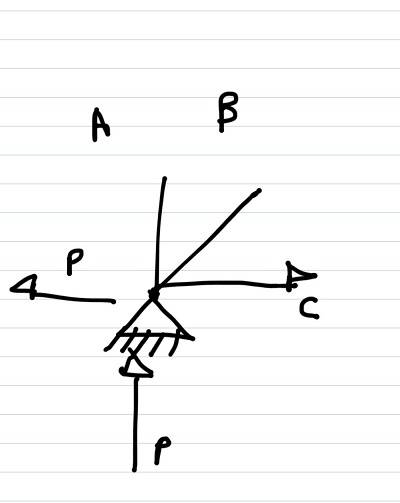
How do you know what direction to make vectors A, B and C face when formulating your equation? I guess its unknowable really isn't it?

therefore for the sum of forces in x direction you'd just say C + P + B_x = 0

How about if it is knowable? E.g. if B didn't exist and therefore you'd know C definitely points in the opposite direction to P_x.
If you then modeled your equation the same as above, and just ignoring signs like this, would you still get the correct answer? P + C = 0
9THChMq.jpg
 
Physics news on Phys.org
I'm having some trouble reading your diagram. What direction are A and B pointing
 
cpsinkule said:
I'm having some trouble reading your diagram. What direction are A and B pointing
My point is that it's unknowable
 
Ry122 said:
My point is that it's unknowable
Yes, it is, given how vaguely worded the OP was.

You mention "your equation", but I haven't the foggiest idea what you are talking about.
 
Okay, I'll reword my question. I guess you just need to make an assumption to begin with about which way each vector faces, right? Let's say A and B both point towards the joint, but this turns out to be wrong. How do you know you were wrong?
 
if your figure is a free body diagram then you need no assumptions. you need to project your known forces onto some axes and use the requirements for static equilibrium ( i assume that is what this problem is) ΣF=0 for each basis direction
 
just take a guess. if your guess is "wrong" you will get a negative number
 

Similar threads

Engineering Loads on a shaft with V-Belts
  • · Replies 2 ·
Replies
2
Views
3K
How to determine direction of a moment
  • · Replies 5 ·
Replies
5
Views
5K
Statics: finding forces in frame
  • · Replies 3 ·
Replies
3
Views
2K
How do I know which direction the horizontal force acts
  • · Replies 4 ·
Replies
4
Views
3K
Force equivalency in a physical simulation
  • · Replies 5 ·
Replies
5
Views
2K
Mastering 3D Statics: How to Solve Moments and Forces for Bearings A and B
  • · Replies 1 ·
Replies
1
Views
3K
To what extent does this system behave as a pendulum?
  • · Replies 1 ·
Replies
1
Views
1K
Solving Beam Deflection with Singularity Equations
  • · Replies 2 ·
Replies
2
Views
2K
Coulombic force of repulsion/attraction between ions
  • · Replies 7 ·
Replies
7
Views
8K
Engineering How Does Cup Anemometer Design Utilize Fluid Mechanics Principles?
  • · Replies 4 ·
Replies
4
Views
3K