bignsud
- 1
- 0
Homework Statement:: Mathematics to understand Quantum Scattering Theory
Relevant Equations:: Suitable math book to understand Quantum Scattering Theory
I need to study Scattering theory from Introduction to Quantum Mechanics by David Griffith. But I think I need to study mathematics first because I only studied: Basic Mathematics, Algebra and Geometry, Calculus, and Differential Equations. I need to study some math but I do not know what book will be suitable for me and what chapters I must study. please help me with some recommendations. Thanks.
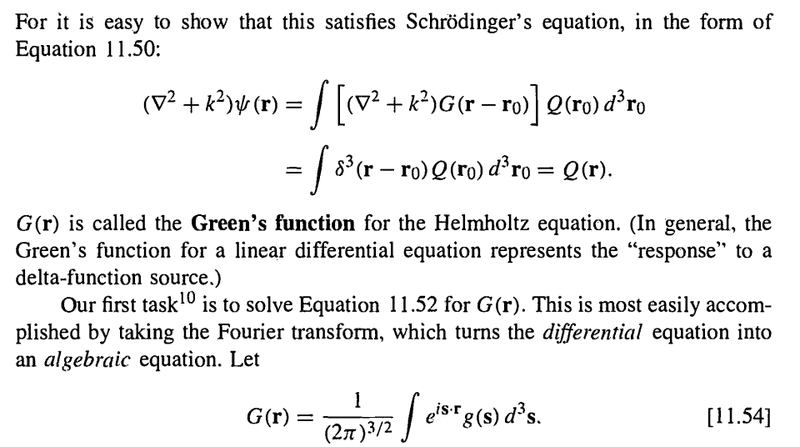
Relevant Equations:: Suitable math book to understand Quantum Scattering Theory
I need to study Scattering theory from Introduction to Quantum Mechanics by David Griffith. But I think I need to study mathematics first because I only studied: Basic Mathematics, Algebra and Geometry, Calculus, and Differential Equations. I need to study some math but I do not know what book will be suitable for me and what chapters I must study. please help me with some recommendations. Thanks.