Ashish Somwanshi
- 31
- 4
- Homework Statement
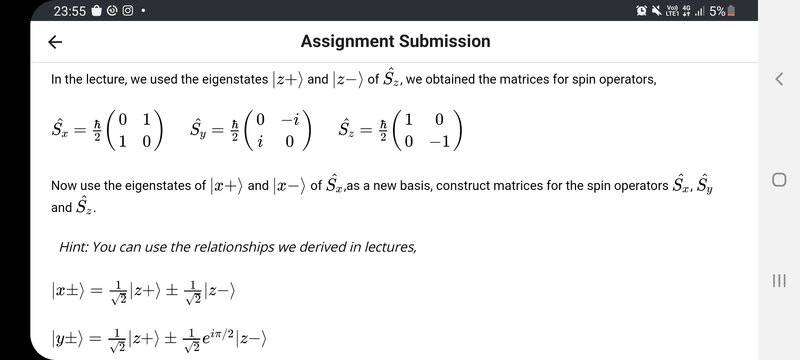
- In the lecture, we used the eigenstates |z+⟩ and |z−⟩ of Sz^, we obtained the matrices for spin operators
Sx^=ℏ/2(0,1,1,0) Sy^=ℏ/2(0,i,−i,0) Sz^=ℏ/2(1,0,0,−1)
note: the numbers in brackets are 2×2 matrices!!!
Now use the eigenstates of |x+⟩ and |x−⟩ of Sx^,as a new basis, construct matrices for the spin operators Sx^, Sy^ and Sz^.
- Relevant Equations
- Both Question and Relevant equations are posted below in attempt.
This screenshot contains the original assignment statement and I need help to solve it. I have also attached my attempt below. I need to know if my matrices were correct and my method and algebra to solve the problem was correct...