- #1
Happiness
- 694
- 31
Let the operators ##\hat{A}## and ##\hat{B}## be ##-i\hbar\frac{\partial}{\partial x}## and ##x## respectively.
Representing these linear operators by matrices, and a wave function ##\Psi(x)## by a column vector u, by the associativity of matrix multiplication, we have
##\hat{A}(\hat{B}##u##)##=##(\hat{A}\hat{B})##u.
By the definitions of ##\hat{A}## and ##\hat{B}##, we have
LHS ##= -i\hbar\frac{\partial}{\partial x}\big(x\ \Psi(x)\big) = -i\hbar\big(x\frac{\partial\Psi(x)}{\partial x} + \Psi(x)\big)##
RHS ##= -i\hbar\Psi(x)##
LHS ##\neq## RHS, a contradiction. Where is the mistake?
The extract from a textbook below only talks about the matrix representation of a Hermitian operator. But is it true that all linear operators can be represented by matrices, not just those that are Hermitian?
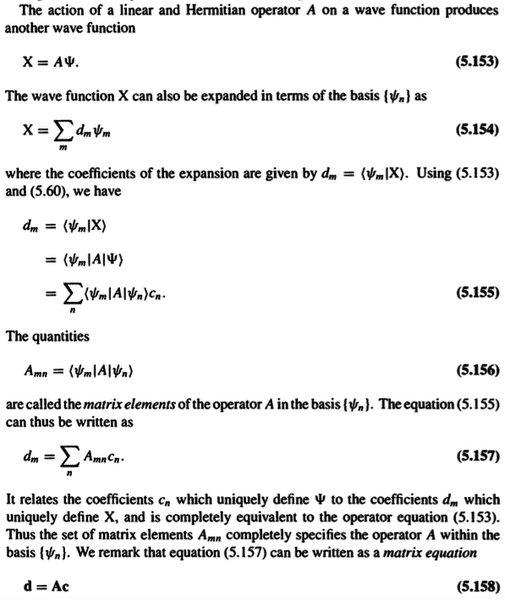
Representing these linear operators by matrices, and a wave function ##\Psi(x)## by a column vector u, by the associativity of matrix multiplication, we have
##\hat{A}(\hat{B}##u##)##=##(\hat{A}\hat{B})##u.
By the definitions of ##\hat{A}## and ##\hat{B}##, we have
LHS ##= -i\hbar\frac{\partial}{\partial x}\big(x\ \Psi(x)\big) = -i\hbar\big(x\frac{\partial\Psi(x)}{\partial x} + \Psi(x)\big)##
RHS ##= -i\hbar\Psi(x)##
LHS ##\neq## RHS, a contradiction. Where is the mistake?
The extract from a textbook below only talks about the matrix representation of a Hermitian operator. But is it true that all linear operators can be represented by matrices, not just those that are Hermitian?