- #1
HelloCthulhu
- 151
- 3
Homework Statement
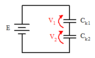
A parallel plate capacitor has area A = 1 cm2 and a plate separation of d = 0.01m. Water at room temp (20°C) is poured into a ziplock bag and placed between the plates filling the volume of 1cm3. Find the maximum capacitance, voltage and charge for the capacitor.
Homework Equations
Q=CV
ziplock:
bag thickness = 1.5mm x 2 (touches both sides of the capacitor = 3mm = 0.003m
dielectric constant = 2.3
dielectric breakdown = 25MV/m
water:
thickness = 0.007m
dielectric constant = 80.1
dielectric breakdown = 65MV/m
Capacitance for two dielectrics in series:
$$C=\frac{\varepsilon_0A}{\frac{d_1}{k_1}\frac{d_2}{k_2}}$$
Voltage for two dielectrics in series:
$${V_r} = {E_{r1}}{d_1} + {E_{r2}}{d_2}$$
The Attempt at a Solution
Maximum capacitance:
$$\frac{(8.85\times10^{-12})\times0.0001}{\frac{0.003}{2.3}+\frac{0.007}{80.1}}=6.35895\times10^{-13}F=0.635895nF=$$Maximum voltage:
$$(65\times10^6V\times0.007m)+(25\times10^6V\times0.003m) =(45.5\times10^3V)+(75\times10^3V) = 455kV+75kV=530kV$$Maximum charge:
$$(6.35895\times10^{-13}F)\times(53\times10^{4}V)=(3.370243\times10^{-7}C)=337.0243nC$$
Last edited: