Maxima and Minima (vector calculus)

[WMDhamnekar]

Hi, Hi,

Author said If we look at the graph of $ f (x, y)= (x^2 +y^2)*e^{-(x^2+y^2)},$ as shown in the following Figure it looks like we might have a local maximum for (x, y) on the unit circle $ x^2 + y^2 = 1.$

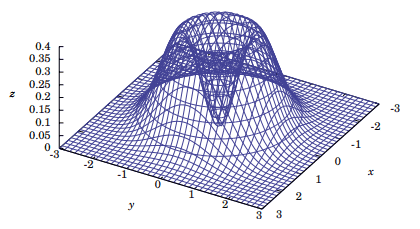But when I read this graph, I couldn't guess that the stated function have a local maximum on the unit circle $ x^2 + y^2=1$

1)I want to know what did author grasp in the above figure which compelled him to make the aforesaid statement?

2) How to plot this function in 'R' or in 'GNU OCTAVE' or in any graphing calculator ? Is it easy to plot $f(x,y)= (x^2+y^2)*e^{-(x^2+y^2)} ?$

 

[Kansas Boy]

Do you notice that the only way x and y appear in that function is as "$x^2+y^2$"? In cylindrical coordinates we can write it as $f(r,\theta)= r^2e^{-r^2}$. If we write it as $y= x^2e^{x^2}$ its graph looks like this: <iframe src="https://www.desmos.com/calculator/oj7v5yfd0f?embed" width="500" height="500" style="border: 1px solid #ccc" frameborder=0></iframe>

Do you see what happens at x= 1 and x= -1? Imagine rotating that around the y-axis.