- #1
anemone
Gold Member
MHB
POTW Director
- 3,883
- 115
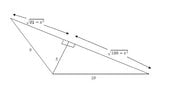
Find the maximum of $P(x)=\dfrac{x(\sqrt{100-x^2}+\sqrt{81-x^2})}{2}$.