Maximum impact parameter given effective potential

[stephen8686]

This problem is from David Morin's classical mechanics textbook:

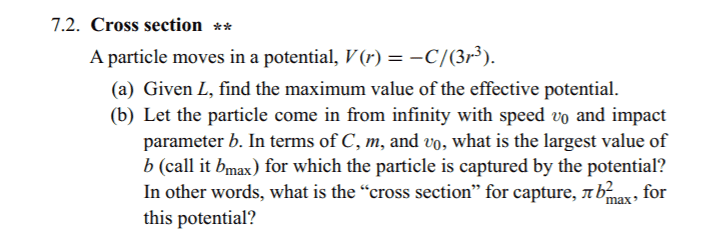
I am having trouble with Part b. Here is the textbook's answer:

I do not understand why large particle energies lead to capture. I would think that smaller energies would lead to capture because the particle wouldn't have enough energy to escape the gravitational potential, whereas large energy particles could woosh past. If someone could explain why my intuition is wrong, that would be very helpful.

 

[PeroK]

How do you make out that larger energies lead to capture?

 

[stephen8686]

How do you make out that larger energies lead to capture?

That's what the answer says, "The condition for capture is therefore $V_{eff}^{max}\leq E$ " That is the part of the answer that I don't understand

 

[PeroK]

That's what the answer says, "The condition for capture is therefore $V_{eff}^{max}\leq E$ " That is the part of the answer that I don't understand

That condition resolves into a smaller impact parameter and smaller cross section for capture for greater energy.

 

[PeroK]

That's what the answer says, "The condition for capture is therefore $V_{eff}^{max}\leq E$ " That is the part of the answer that I don't understand

That equation in itself is about the relationship between angular momentum and energy. But, angular momentum increases with energy if other factors are held constant, so it doesn't say what you are thinking it says.