- #1
Mikkel
- 27
- 1
- Homework Statement
- Measure distance using magnitude and extinction
- Relevant Equations
- m - M = 5log_{10}(d/10 pc) + 10^{-3}
Hello, I'm struggling with this question
I think I got the generel idea right for this problem, but I must be messing up my SI units somewhere. I use the distance formula when working with magnitudes and extinction. I look up the absolute magnitude for a type A3 star, which is roughly 2. I solve for d and end up with a distance of 398kpc, which doesn’t make sense, as the star is at the center of the Milky Way.
I use this formula and I divide A by 10^3 to get units of pc.
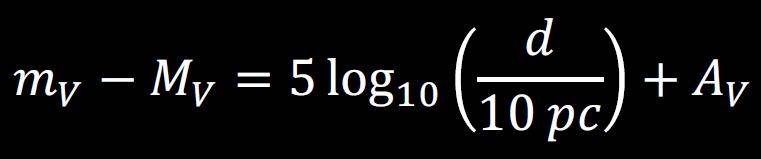

I hope someone can spot my mistake
- A star is observed close to the center of the Milky Way and from its spectrum we find that it is a type A3 star. Its observed magnitude is m_v = 25. There is only a diffusive gas between us and the star, so we can assume an extinction, of 1 magnitude per 1 kpc.
- How far away is the star and what is its (un-redded) magnitude?
- In the H-band, which is 1.63 , the reddening law is . How big is the reddening in the H-band?
I think I got the generel idea right for this problem, but I must be messing up my SI units somewhere. I use the distance formula when working with magnitudes and extinction. I look up the absolute magnitude for a type A3 star, which is roughly 2. I solve for d and end up with a distance of 398kpc, which doesn’t make sense, as the star is at the center of the Milky Way.
I use this formula and I divide A by 10^3 to get units of pc.
I hope someone can spot my mistake