Measuring Magnetic Field in Rotating Frame

[Xynon]

Consider that we have a magnet and a magnetometer (a fluxgate magnetometer with a single coil), standing still as shown in fig 1.

In fig 1, the magnetic field measured at the axis z₁ of the magnetometer coil is B₁.

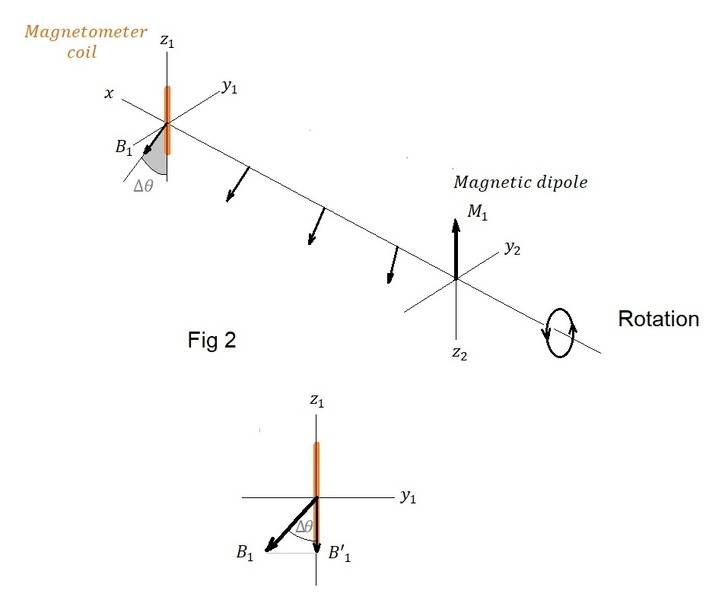
But if everything (magnet, magnetometer and the axes) was rotating together around the x-axis as in fig 2, would there be a decrease in the measured magnetic field on the coil axis z₁ due to the retarded angular position ∆θ? In other words, a tilt in the opposite direction of the rotation which decreases the component of B₁ on the coil axis z₁ ?

If so, could we state that B'₁=B₁ cos (∆θ) ?

Can we say that this angle of tilt ∆θ is a phase shift, if we consider the rotating magnet as a transmitting and the coil as a receiving antenna?

 

[pervect]

If the frame rotates around the dipole, labelled M1 on the diagram, there will be an effect on the reading of coil, B1, due to the motion of the coil due to the velocity of B1 induced by the rotation. I haven't worked out what this effect will be, exactly.

If I understand the problem correctly, in the non-rotating frame the E field is zero everywhere. This will basically specify what we mean by the non-rotating frame, it's the only frame where the E-field is zero everywhere. It will also be the only inertial frame. Therefore, if we rely on high-school/undergraduate physics methods that only work in inertial frames, that's the frame we solve the problem in.

The most concise description of this approach is to say that the combined B and E field are the components of a rank-2 tensor, the so-called Faraday tensor, and that the tensor nature of the Faraday tensor defines how it must transform. The tensor description implies by the definition of how tensors transform that only velocities matter, not rotations or accelerations.

The tensor approach gives us the freedom to solve the problem in one frame, and transform the solution to another frame, including non-inertial rotating frames.

However, if one is not familiar with tensors, this concise description may not help. My best answer in that case is to say that the problem specification defines a specific frame where the E-field is zero, and we work out the solution to the problem in that frame. Without tensors, one is generally taught to re-solve the problem in every new frame. There might be some discussion of how solutions in one frame imply solutions in other frames without tensors, but I'm not aware of where one might find a discussion that doesn't involve tensors.

There is probably a better high-school/undergraduate level approach , but I don't know what it is. We do have discussions of Newtonian physics in rotating frames at this level, so why can't we solve this dipole in-a-rotating frame problem at this level? I suspect that one of the issues here is that Maxwell's equations are essentially relativistic rather than Newtonian, and that this is the ultimate stumbling block here. But that observation isn't high-school/undergraduate level either.

 

[sweet springs]

If so, could we state that B'1=B1 cos (∆θ) ?

Yes. In IFR where the magnetic dipole is rotating, it takes time for the dipole position signal to reach measuring point.
In rotation system geometry change or curved geodesic would explain the phenomena though I have not been into detail.

f I understand the problem correctly, in the non-rotating frame the E field is zero everywhere. This will basically specify what we mean by the non-rotating frame, it's the only frame where the E-field is zero everywhere. It will also be the only inertial frame. Therefore, if we rely on high-school/undergraduate physics methods that only work in inertial frames, that's the frame we solve the problem in.

Circuit current which generates magnetic dipole is bipolar charged by Lorentz transformation of tangent speed by rotation. These charges generate electric field. See formula (13.24), (13.25) and (13.26) in Feynman http://www.feynmanlectures.caltech.edu/II_13.html
I am not sure thus generated electric field has something to do with OP's question at least directly.

 

[pervect]

If the frame rotates around the dipole, labelled M1 on the diagram, there will be an effect on the reading of coil, B1, due to the motion of the coil due to the velocity of B1 induced by the rotation.

I need to clarify/ammend this. If B1 and B2 are two measuring instruments at the same point in space, and if B1 is at rest in the non-rotating frame, and B2 is at rest in the rotating frame, then B1 and B2 have different velocities. B1 and B2 also differ in a relative rotation, though we can assume that the axes of B1 and B2 align at that instant of time in which they co-incide.

By "rotating frame", I mean a frame rotating around the source at M1, as I mentioned in the previous post.

The velocity difference between B1 and B2 causes differences in their readings. The rotation about the origin doesn't cause any differences in readings _at the origin of rotation_.