srnixo
- 51
- 10
- Homework Statement
- .
- Relevant Equations
- ,
Here is the exercise:
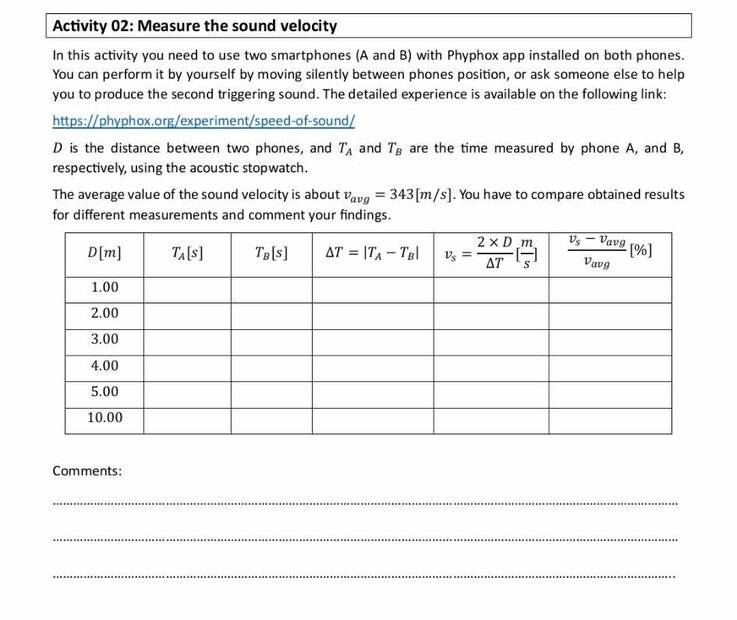
[ Normally when we increase distances, the velocity of sound decreases?]
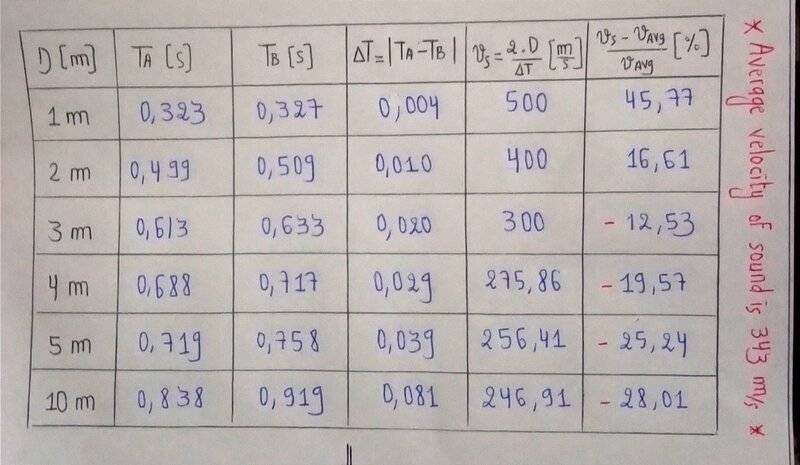
Or this one?
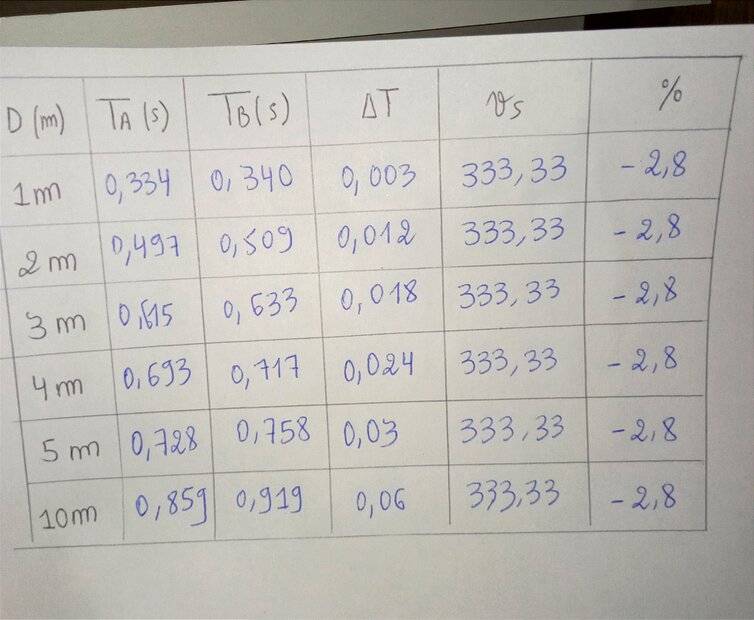
You might wonder why. Well, my friend in class told me that the second table could be correct because the experiment was conducted at closed home under suitable conditions, where the average speed of sound is around 343 and the calculated speed is approximately 333, which is slightly lower than the standard value but still within a reasonable range of deviation so somewhat acceptable within the limits!
and she told me also that in home, when we increase distances, the velocity of sound remains constant. This is because the speed of sound in a medium (such as air) is determined by the properties of that medium, and not by the distance traveled within it.
could you please help me!
Which one seems logical and correct ?
this one:[ Normally when we increase distances, the velocity of sound decreases?]
Or this one?
You might wonder why. Well, my friend in class told me that the second table could be correct because the experiment was conducted at closed home under suitable conditions, where the average speed of sound is around 343 and the calculated speed is approximately 333, which is slightly lower than the standard value but still within a reasonable range of deviation so somewhat acceptable within the limits!
and she told me also that in home, when we increase distances, the velocity of sound remains constant. This is because the speed of sound in a medium (such as air) is determined by the properties of that medium, and not by the distance traveled within it.
could you please help me!