Mechanics of Materials questions

[i-love-physics]

Hello Everyone

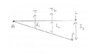
I have a mechanics of materials questions which I have been stuck on for hours, I just can't figure it out.

Attached is the questions.


I know the formula for deflection which is PL/ AE

but what do i do with that formula in this situation?

Any help would be greatly appreciated.

Thanks!

 

[minger]

The first thing you need to do is find the reactions of the supports. How are you coming with that?

 

[i-love-physics]

The first thing you need to do is find the reactions of the supports. How are you coming with that?

The problem is that there are too many unknowns when I try and find the reactions.

For example

If I take moments at A = 0 Then Force CD is a unknown and Force EF is a unknown

IF i take moments at C = 0 Then force Ay is unknown and Force Ef is unknown

I am stuck :(

 

[Studiot]

Do we really need the reaction at A?

In the diagram by similar triangles

$$\frac{{{\delta _2}}}{{{\delta _1}}}\quad = \quad ?$$

This means

$$\frac{{{T_2}}}{{{T_1}}}\quad = \quad ?$$

Since the hinge forces do not move they do no work

Equating work done by load to Strain work done by tensions in rods

$$L{\delta _3}\quad = \quad {T_1}{\delta _1}\quad + {\kern 1pt} \quad {T_2}{\delta _2}$$

I have not done it for you but you should be able to figure it out from here.

 

[i-love-physics]

Do we really need the reaction at A?

In the diagram by similar triangles

$$\frac{{{\delta _2}}}{{{\delta _1}}}\quad = \quad ?$$

This means

$$\frac{{{T_2}}}{{{T_1}}}\quad = \quad ?$$

Since the hinge forces do not move they do no work

Equating work done by load to Strain work done by tensions in rods

$$L{\delta _3}\quad = \quad {T_1}{\delta _1}\quad + {\kern 1pt} \quad {T_2}{\delta _2}$$

I have not done it for you but you should be able to figure it out from here.

Thanks for the help

so pretty much s2/s1 = t2/t1 and L*s3 = t1*s1 + t2*s2

k so how do i figure out what s3 is??

as the formula for deflection is (T)(L) / (E)(A) for s3 what would the L be? and what would the e and a be?

I am still lost :(

 

[pongo38]

L A and E are properties of the rods CD and EF. The question gives these (or enough to compute them anyway)

 

[i-love-physics]

L A and E are properties of the rods CD and EF. The question gives these (or enough to compute them anyway)

ok so if s3 = (25000)*(1500) / (70000)*(490.87) then = 1.091 mm

so what do I do now to find t1 and t2?

 

[Studiot]

You said earlier that there were too many unknowns, which implies that there were not enough equations.

I offered you some equations; you can get some more by the same methods.

What do you normally do with a bunch of equations?

Hint try solving the first one I gave you, you don't seem to have done that yet.

 

[i-love-physics]

You said earlier that there were too many unknowns, which implies that there were not enough equations.

I offered you some equations; you can get some more by the same methods.

What do you normally do with a bunch of equations?

Hint try solving the first one I gave you, you don't seem to have done that yet.

I found the answer to the questions.

I used principles of superposition. You could have told me to just read about principles of superposition but you probably had no clue yourself.

Thanks for trying though.

 

[Studiot]

The solution I offered you does not involve superposition.

By using the geometry of the situation, (similar triangles) you can obtain

$${\delta _2}$$ and $${\delta _3}$$ in terms of $${\delta _1}$$

Using the elastic relations (hookes law) you can obtain $${T_2}$$ in terms of $${T_1}$$

Then you can substitute into my third equation which is a simple energy method.
You then have a single equation in terms of one unknown $${T_1}$$.
The rest follows.


I'm glad you eventually found your own solution to what appears to be a homework question.

 

[Studiot]

Whilst superposition is an important idea, the one thing to take away form this is called compatibility.

Following equilibrium the next most important euqations in the armoury are the equations of compatibility.

These are derived from purely geometric considerations about the situation and are more gnerally and widely applicable than superposition.

In this particular example compatibility requires that the deflections are in the sameratio as the lengths along the beam.