mhrob24
- 53
- 9
Summary:: Torsional stress on freely spinning shaft?
Hey guys,
I’m having some confusion with a certain section of the “Torsion” chapter in my mechanics of materials book: “power transmission”.
Please see the problem below. This is very easy to SOLVE (basically plug and chug with the equations), but conceptually, I am not understanding something:
I do not see how we are allowed to use the torsional formula to solve for the minimum shaft DIA when from what I see, there is no TORSIONAL stress. The problems that were explained earlier in the chapter made sense because they were FIXED at one end. So the support fights the rotation, and because of this, the polar moment of inertia due to the shaft geometry is fighting torsional deformation. Without the support, the way I see it currently, the polar moment of inertia isn’t really “fighting” anything…right?
Like, when I look at this: all I see stress-wise is a shear stress due to reaction forces from the “supports” (the motor attachment point and the bearing support) that prevent translation of the shaft, and the shear stress from the tension force of that belt (but it’s not given, so it’s assumed zero). I know it doesn’t say to solve for torsional stress, but we’re using the torsional stress formula to solve it.
Idk where I’m going wrong here, but I’m spending too much time thinking about this now….
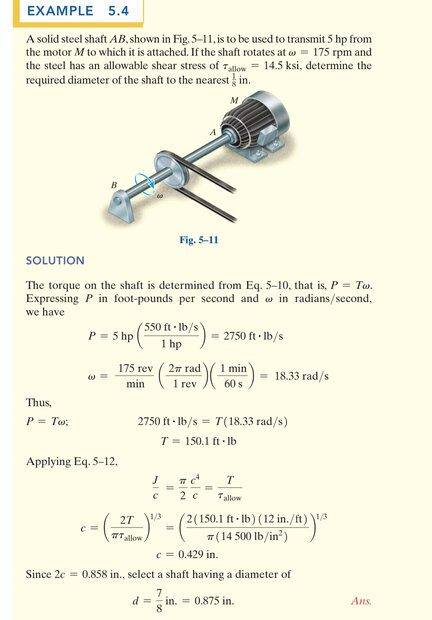
Hey guys,
I’m having some confusion with a certain section of the “Torsion” chapter in my mechanics of materials book: “power transmission”.
Please see the problem below. This is very easy to SOLVE (basically plug and chug with the equations), but conceptually, I am not understanding something:
I do not see how we are allowed to use the torsional formula to solve for the minimum shaft DIA when from what I see, there is no TORSIONAL stress. The problems that were explained earlier in the chapter made sense because they were FIXED at one end. So the support fights the rotation, and because of this, the polar moment of inertia due to the shaft geometry is fighting torsional deformation. Without the support, the way I see it currently, the polar moment of inertia isn’t really “fighting” anything…right?
Like, when I look at this: all I see stress-wise is a shear stress due to reaction forces from the “supports” (the motor attachment point and the bearing support) that prevent translation of the shaft, and the shear stress from the tension force of that belt (but it’s not given, so it’s assumed zero). I know it doesn’t say to solve for torsional stress, but we’re using the torsional stress formula to solve it.
Idk where I’m going wrong here, but I’m spending too much time thinking about this now….