- #36
Leb
- 94
- 0
I am still not sure if this all helped (not that your explaining is bad, but me being daft). I am now looking at shocks and weak solutions. I (think I) know how to mechanically get weak solutions, but when it comes to drawing the characteristics for the weak solution - I'm in trouble.
For example here:
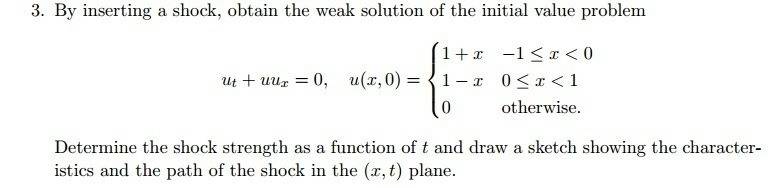
solution:
I can sort of understand why it is straight lines before -1 and after 1 (from below). Because I think I should be looking for the gradient (1/c(σ)) and c(σ) = ρ(x,t), so the 1/0 gives and infinite gradient (i.e. a straight line), but what about the non straight lines ? How to I look at
1+t/(1+x) ? I would probably try and set t first, so the -1<x<sqrt would hold (for example, t = 1) and then just pick any x from those values (in this case x is in (-1;1).
I am also not sure where the "fan" (chars from 0 to 1 point to (1,1)) originates (it's not in the weak solution, or at least I cannot see it...
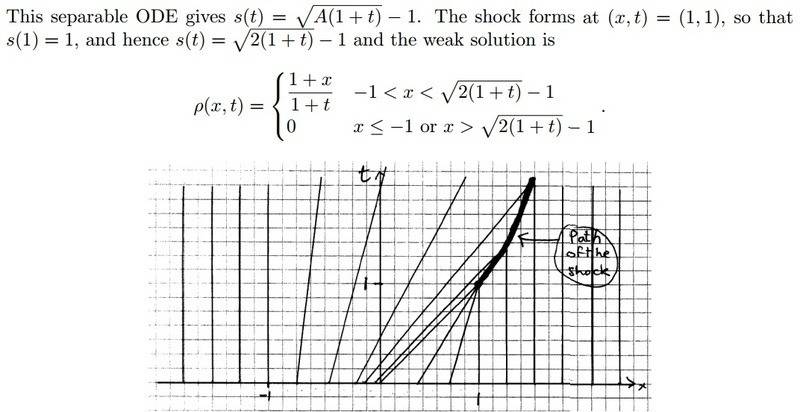
For example here:
solution:
I can sort of understand why it is straight lines before -1 and after 1 (from below). Because I think I should be looking for the gradient (1/c(σ)) and c(σ) = ρ(x,t), so the 1/0 gives and infinite gradient (i.e. a straight line), but what about the non straight lines ? How to I look at
1+t/(1+x) ? I would probably try and set t first, so the -1<x<sqrt would hold (for example, t = 1) and then just pick any x from those values (in this case x is in (-1;1).
I am also not sure where the "fan" (chars from 0 to 1 point to (1,1)) originates (it's not in the weak solution, or at least I cannot see it...