Method of Images, combination of an infinite plane and a hemisphere

[milkism]

Homework Statement

Find the surface density on the conducting surface in terms of potential either in cartesian coordinates or spherical.

Relevant Equations

See solution.

Problem:

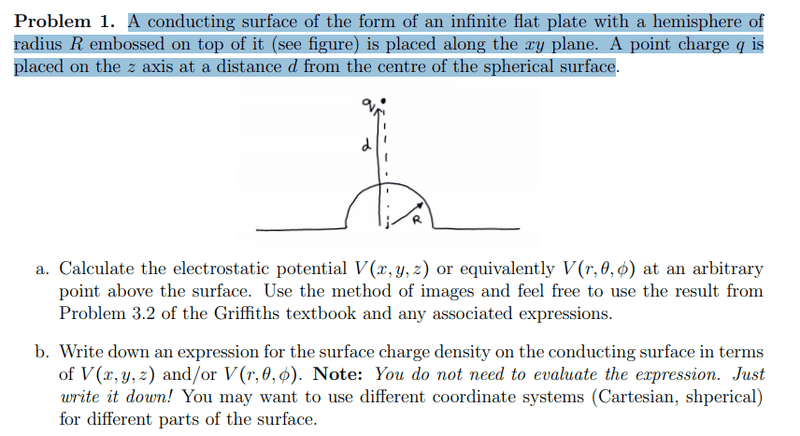
I have done part a) in spherical polar coordinates.
For part b) I thought it would be just:
$$\sigma = -\epsilon_0 \frac{\partial V}{\partial r}$$
But I got confused by "You may want to use different coordinate systems .." So I assume partial derivative w.r.t to r is the spherical part, what would the cartesian part be? I assume for the cartesian part it would be the partial derivative with respect to z, but in my solution for part a) I don't have a z-component, do I have to find the potential in Cartesian coordinates also?

 

[PhDeezNutz]

is there an expression for gradient in spherical coordinates on the back cover of Griffiths?

I’d evaluate the gradient of the expression from part a) generally and then set

$\theta = \frac{\pi}{2}$

And of course take the dot product with z-hat

There’s an expression on the back cover of Griffiths for z-hat in spherical basis as well.

 

[milkism]

is there an expression for gradient in spherical coordinates on the back cover of Griffiths?

I’d evaluate the gradient of the expression from part a) generally and then set

$\theta = \frac{\pi}{2}$

And of course take the dot product with z-hat

There’s an expression on the back cover of Griffiths for z-hat in spherical basis as well.

So basically $$z=r\cos(\theta)$$. I put that in my potential in spherical coordinates then
$$\sigma = -\epsilon_0 \left( \frac{\partial V}{\partial r} \cdot \frac{\partial V}{\partial z} \right)$$
?

 

[PhDeezNutz]

The gradient is always perpendicular to the equipotential. So you’re going to have to break this problem into two parts. Finding the surface charge on the curved part and then the flat part. Do them separately.

1) for the curved part $\frac{\partial V}{\partial r}$ will do just fine

2) for the flat part you’re going to have to get more general $\sigma = - \nabla V \cdot \hat{z}$.

When you evaluate $\nabla V$ you’ll get something in spherical basis (the second expression)

We have to express $\hat{z}$ in spherical basis

Evaluate the dot product generally then plug in theta is pi/2.

 

[milkism]

The gradient is always perpendicular to the equipotential. So you’re going to have to break this problem into two parts. Finding the surface charge on the curved part and then the flat part. Do them separately.

1) for the curved part $\frac{\partial V}{\partial r}$ will do just fine

2) for the flat part you’re going to have to get more general $\sigma = - \nabla V \cdot \hat{z}$.

When you evaluate $\nabla V$ you’ll get something in spherical basis (the second expression)

View attachment 325229

We have to express $\hat{z}$ in spherical basis
View attachment 325231

Evaluate the dot product generally then plug in theta is pi/2.

How did you come up with the equation for the flat part? I have never seen it, and shouldn't there also be an epsilon_zero?

 

[PhDeezNutz]

I didn’t come up with it. It’s a definition.

The “normal derivative” literally means “directional derivative in the direction of the normal”. Directional derivative is defined by the dot product of the gradient with the direction unit vector of interest”. For the flat part the direction of interest is z-hat (because that is the normal).

And of course there should be a $-\epsilon_0$ in front. I was being lazy.

 

[milkism]

I didn’t come up with it. It’s a definition.

The “normal derivative” literally means “directional derivative in the direction of the normal”. Directional derivative is defined by the dot product of the gradient with the direction unit vector of interest”. For the flat part the direction of interest is z-hat (because that is the normal).

And of course there should be a $-\epsilon_0$ in front. I was being lazy.

Thanks!

 

[milkism]

I didn’t come up with it. It’s a definition.

The “normal derivative” literally means “directional derivative in the direction of the normal”. Directional derivative is defined by the dot product of the gradient with the direction unit vector of interest”. For the flat part the direction of interest is z-hat (because that is the normal).

And of course there should be a $-\epsilon_0$ in front. I was being lazy.

Last question should the total induced charge on the conducting surface be -q, or $$q' = \frac{-qR}{d}$$? If it was just a flat surface it would have been -q, if it was just a sphere it would have been the latter.

 

[PhDeezNutz]

Last question should the total induced charge on the conducting surface be -q, or $$q' = \frac{-qR}{d}$$? If it was just a flat surface it would have been -q, if it was just a sphere it would have been the latter.

I’m not sure. I’d have to work it out.

At the very least it’s not going to be q.

 

[milkism]

I’m not sure. I’d have to work it out.

At the very least it’s not going to be q.

Well, question asked to find the charge without doing any calculation, because we didn't have to calculate the surface charge density either (we just had to give an expression), I only know that it will be a negative charge haha

 

[haruspex]

question asked to find the charge without doing any calculation

I assume that in the first part you came up with three point charges to represent the induced charge distribution. Is the total induced charge simply their sum?

Btw, I note the text of the question says q is at d from the spherical surface, but your diagram has it at d from the centre of curvature.