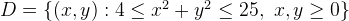Petrus
- 702
- 0
Hello MHB,
I got as homework to solve this problem and get recommend to solve it with polar but I have not really work with polar but we have had lecture about it and I have done some research. This is the problem and what I understand
$$\int\int_Dx^3y^2\ln(x^2+y^2)$$, $$4\leq x^2+y^2\leq 25$$ and $$x,y\geq 0$$
if we want to change it to polar form let's write $$x=r\cos\theta$$ and $$y=r\sin\theta$$
so we got:
$$\int_{\theta_1}^{\theta_2}\int_{r_1}^{r_2}r^3\cos^3\theta*r^2\sin^2\theta \ln(r^2\cos^2\theta+r^2\sin^2\theta) \ drd\theta$$
and we got our identity that $$x^2+y^2=r^2$$ that means we got our r limit as $$2\leq r \leq 5$$ if I am thinking correct we can't use our negative limit cause it says $$x,y\geq 0$$ I am stuck with how to get my $$\theta$$ limit well so far I can think we know that $$4 \leq r^2cos^2\theta + r^2sin^2\theta \leq 25$$ here is what I strugle with. is solve limit for $$\theta$$
Regards,
I got as homework to solve this problem and get recommend to solve it with polar but I have not really work with polar but we have had lecture about it and I have done some research. This is the problem and what I understand
$$\int\int_Dx^3y^2\ln(x^2+y^2)$$, $$4\leq x^2+y^2\leq 25$$ and $$x,y\geq 0$$
if we want to change it to polar form let's write $$x=r\cos\theta$$ and $$y=r\sin\theta$$
so we got:
$$\int_{\theta_1}^{\theta_2}\int_{r_1}^{r_2}r^3\cos^3\theta*r^2\sin^2\theta \ln(r^2\cos^2\theta+r^2\sin^2\theta) \ drd\theta$$
and we got our identity that $$x^2+y^2=r^2$$ that means we got our r limit as $$2\leq r \leq 5$$ if I am thinking correct we can't use our negative limit cause it says $$x,y\geq 0$$ I am stuck with how to get my $$\theta$$ limit well so far I can think we know that $$4 \leq r^2cos^2\theta + r^2sin^2\theta \leq 25$$ here is what I strugle with. is solve limit for $$\theta$$
Regards,