Michelson interferometer fringes

[Goodver]

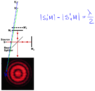
Since 2 splitted beams meet at ONE spot later on, we have just one beam which flows to the detector, therefore I expect to see just one light spot, where the BRIGHTNESS is changing depending on at which phase 2 beams meet. Pattern with fringes happens when we have multiple sources, like Young's experiment or difraction grating.

why we have fringes here if there is only one source?

 

[jtbell]

Indeed, if you set up the interferometer using a narrow laser beam that produces only a single spot on the screen when the mirrors are aligned properly, we see what you describe: a single spot that changes brightness as you change one of the path lengths by moving one of the mirrors back and forth.

To see the "bullseye" pattern of fringes, you have to diverge the beam by placing a lens in front of the laser.

 

[Goodver]

Thanks jybell! I am still confused thought, since there is not diffraction, how come such a pattern can occur? If you diverge the beam, it becomes just less intense right?

 

[jtbell]

since there is not diffraction, how come such a pattern can occur?

Are you thinking of the circular-aperture diffraction pattern?

http://hyperphysics.phy-astr.gsu.edu/hbase/phyopt/cirapp2.html#c1

The circular pattern in the Michelson interferometer is not from diffraction. As you move away from the center of the pattern, the difference between the path lengths for the light rays arriving via the two mirrors changes, and so does the phase difference. You can see this even with a single laser-spot on the screen, if you tilt both of the mirrors slightly so the spot moves away from the center of the pattern, while keeping the two reflected spots together.

If you expand the beam so you can see the entire pattern, and then move one of the mirrors gradually: the rings either expand gradually, with new rings being "created" at the center; or they contract gradually, with rings "disappearing" at the center. The center of the pattern also changes its position.

 

[Goodver]

is my drawing correct? So we expend the beam, that the pattern widens as we move the screen further, thus from two source images the distance to particular spot differs as we go to the side from the center? (i hope you know what i mean)

does this means, that again if we put 2 mirrors at equal disances, two source images get close and merge => when the distances to the mirrors are equal we see just tne solid spot without rings?

 

[jtbell]

is my drawing correct? So we expend the beam, that the pattern widens as we move the screen further, thus from two source images the distance to particular spot differs as we go to the side from the center? (i hope you know what i mean)

Yes, I think you've got it about right. Let the two "virtual sources" be separated by distance d = m₀λ (where m₀ is an integer), and let the distance to the screen be L. It's a nice exercise to derive the radius r of the m'th ring from the center. (Assume r << L so you can use some simplifying approximations.)

does this means, that again if we put 2 mirrors at equal disances, two source images get close and merge => when the distances to the mirrors are equal we see just tne solid spot without rings?

Basically, yes. You have complete constructive interference. The size of the "spot" is limited only by the geometry of your system: the size of the mirrors and maybe other things.

 

[Goodver]

Thank you jtbell!