Sunwoo Bae
- 60
- 4
- Homework Statement
- Shown in the text
- Relevant Equations
- Q = CV
capacitors in series
capacitors in parallel
The following is the question and the solution to the question.
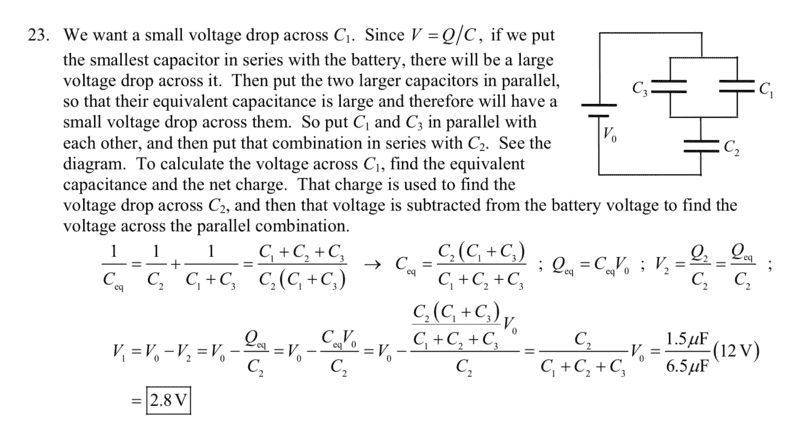
I understand the solution to the part where you find the Ceq and derive Qeq from the equation Q = Ceq*V.
However, I do not understand where V1 = V0-V2 come from.
When calculating the minimum voltage, how do you come up with the equation V1 = V0-V2, and why is V3 not taken to account?
I understand the solution to the part where you find the Ceq and derive Qeq from the equation Q = Ceq*V.
However, I do not understand where V1 = V0-V2 come from.
When calculating the minimum voltage, how do you come up with the equation V1 = V0-V2, and why is V3 not taken to account?