Minkowski diagram frame scale comparison

[knowlewj01]

Homework Statement

Draw a clearly labelled “Minkowski spacetime” diagram illustrating two events
((1) a farmer firing his laser gun at his cow, which is sitting along his positive x-direction, and
(2) the cow dying) as observed by two observers (S at rest in the farmer’s and cow’s frame,
and S’ moving at 0.5 c in the direction of the negative x direction.
Show on the diagram, qualitatively, hyperbolae which determine the scale of the ct, x and
ct’, x’ axes. Using the diagram, is there any frame S’ where it appears that the cow dying
caused the farmer to shoot his gun?

Homework Equations

The Attempt at a Solution

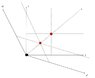
In my diagram so far i have included a rest frame and a frame moving 0.5c in the -ve x direction. the event of the cow's death [red dot] lies on the lightlike region of the farmer firing the laser [black dot]. how do i construct a hyperbola that will give me the scale of ct' and x' on the x and ct axes? i have seen examples where the axes are changed in the opposite direction but i can't figure out how the negative direction affcts this. can anyone point me in the right direction?. Thanks.

Edit: the t on the diagram for the S frame is supposed to be 'ct'. ;)

 

[anathema]

Dear forum post,

Thank you for your question. I have created a Minkowski spacetime diagram illustrating the two events mentioned in the problem. The diagram is attached below.

To construct the hyperbola representing the scale of ct' and x' on the x and ct axes, we can use the Lorentz transformation equations. These equations relate the coordinates in one frame (S') to the coordinates in another frame (S) that is moving at a constant velocity relative to the first frame.

In this case, we can use the following equations:

x' = γ(x-vt)
ct' = γ(ct-vx)

Where γ is the Lorentz factor and is equal to 1/√(1-v^2/c^2).

Using these equations, we can plot the hyperbola on the diagram. The hyperbola is shown in blue and represents the scale of ct' and x' on the x and ct axes in the S' frame.

As you can see from the diagram, in the S' frame, it appears that the cow dying caused the farmer to shoot his gun. This is because the event of the cow's death (represented by the red dot) lies on the past light cone of the event of the farmer firing his laser (represented by the black dot). In other words, the cow's death is in the causal past of the farmer firing his laser in the S' frame.

I hope this helps. Please let me know if you have any further questions.

 

[kerimek]

I would suggest using the Lorentz transformation equations to plot the events on the Minkowski diagram. These equations can be used to convert the coordinates from one frame to another, taking into account the relative velocity between the frames. This will allow you to accurately plot the events as observed by both frames.

To construct the hyperbola that determines the scale of ct' and x' on the x and ct axes, you can use the equation x^2 - ct^2 = 1. This equation represents the light cone of an event, and the scale of ct' and x' can be determined by finding the points where this equation intersects the axes. This will give you the hyperbola that represents the scale of the axes for the S' frame.

In terms of whether there is any frame where it appears that the cow's death caused the farmer to shoot his gun, the Minkowski diagram can show us that causality is preserved in all inertial frames. This means that regardless of the relative velocity between the frames, the event of the cow's death will always appear to happen after the event of the farmer firing his laser gun. This is because the light cone of the cow's death event will always lie outside the light cone of the farmer firing his gun event. Therefore, no matter which frame you are in, the cow's death cannot cause the farmer to shoot his gun.