Physics Forums: Science Discussion, Homework Help, Articles

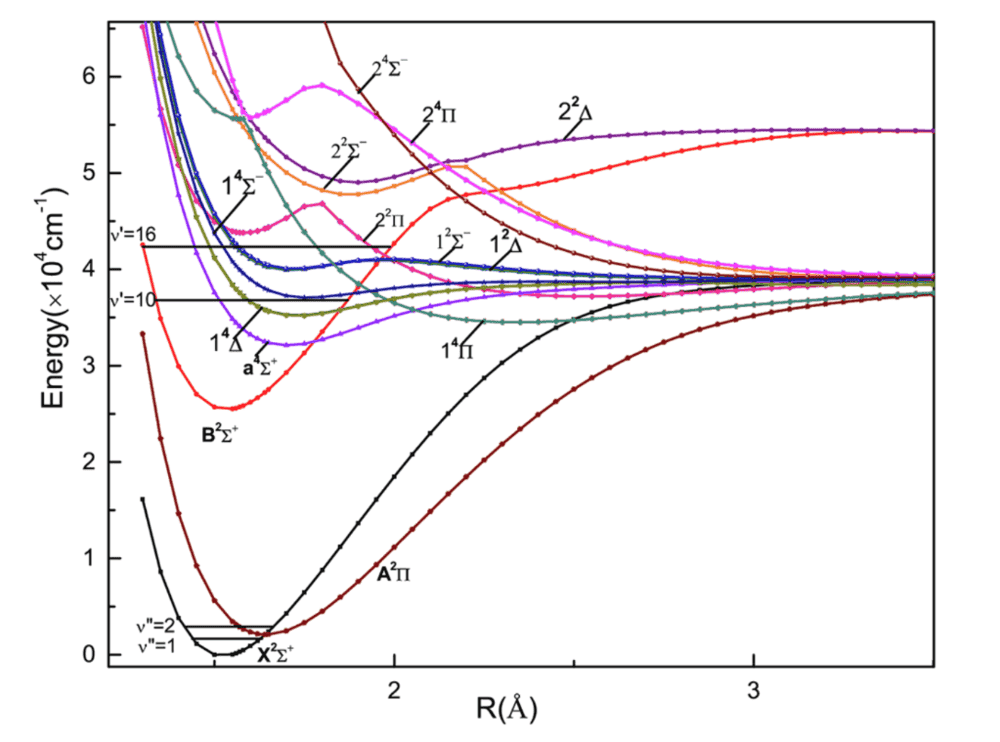
Hello! I am trying to do some molecular physics calculations, involving the calculation of the expectation value of certain vector operators (such as the electric dipole moment of the molecule) in given molecular states. In most cases assuming the Born-Oppenheimer (or adiabatic) approximation makes things easier, as I can separate the rotating frame of the molecule from the lab frame and some terms can be calculated analytically while others are taken from measurements or numerical calculations. However my (limited) experience with these calculations (and basically all the books and papers I read) assumed the Born-Oppenheimer approximation. However now I need to do some calculations in a system whose energy levels look like in the figure below. As you can see the ground state (##X^2\Sigma^+)##, which is what I care about, is very close in energy to the first excited state (##A^2\Pi##). From what I read, the Born-Oppenheimer approximation holds well when the electronic level of interest is separated from the others (so that the higher order corrections going like ##1/\Delta E## are very small). However in our case that is not really the case, so I am not sure I can just use the techniques that I would normally use and I don't really know what to do. Can someone point me towards some books or papers that actually describe this situation? Thank you!