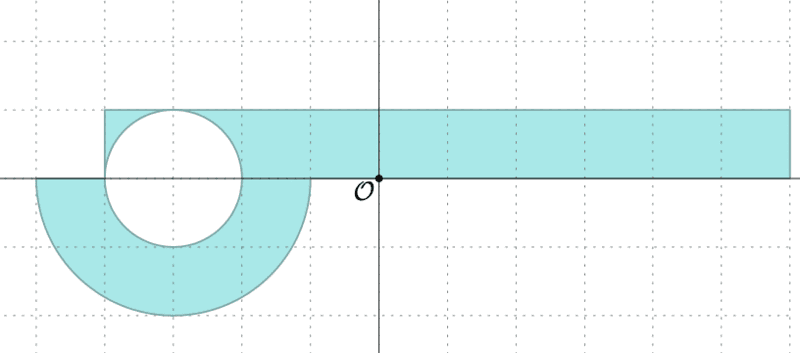
- #1
antonov
- 5
- 2
- Homework Statement
- Marginal of 0,5% is OK
- Relevant Equations
- see picture
I have this moment of inertia problem and is a little confused on the semicircle part and if the rest is really right? I get over 10 if I calculate it in crew CAD but by hand I get 7,568032142. What is right and what am I doing wrong?