Aurelius120
- 269
- 24
- Homework Statement
- Calculate the Moment of Inertia of Disc about axis passing through its center and perpendicular to plane
- Relevant Equations
- $dI=\int{x^2 dm }$
In deriving the MOI of a ring about its center perpendicular to plane, our teacher said think of ring as made up of ##dm## units each at ##R## distance from the axis therefore the MOI becomes: $$I=R^2\int{dm}=MR^2$$
If disc is considered to be made up of rods of ##dx## thickness, in this manner
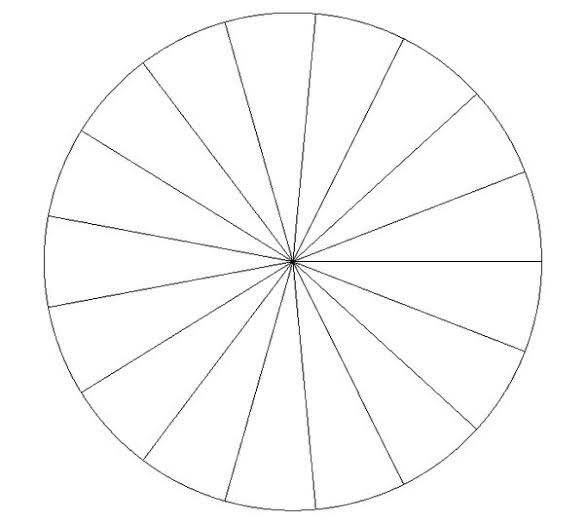
then by the former logic its MOI should be$$I=R^2\frac{\int{dm}}{3}$$ However the correct method of derivation using unequal rods or concentric rings shows that it is $$I=\frac{MR^2}{2}$$ Doesn't it seem paradoxical??
If disc is considered to be made up of rods of ##dx## thickness, in this manner
then by the former logic its MOI should be$$I=R^2\frac{\int{dm}}{3}$$ However the correct method of derivation using unequal rods or concentric rings shows that it is $$I=\frac{MR^2}{2}$$ Doesn't it seem paradoxical??