simphys
- 327
- 46
- Homework Statement
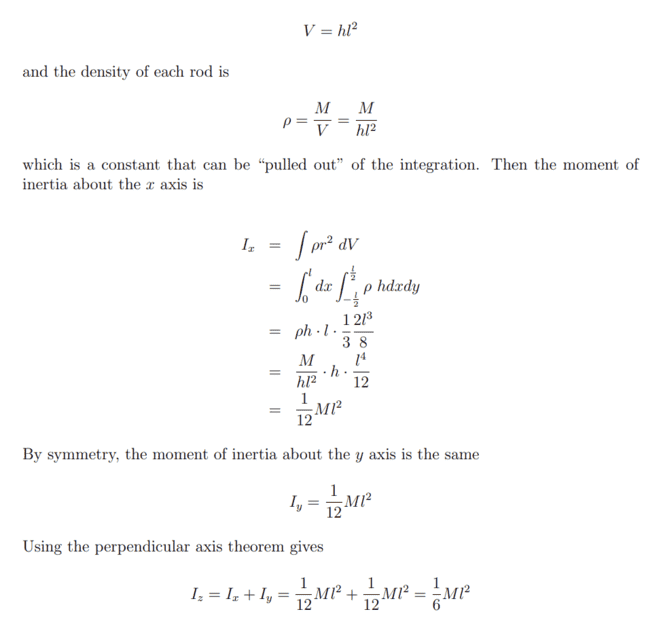
- the moment of inertia about an axis through the center of and perpendicular to a uniform, thin square plate. mass M and dimension L x L.
- Relevant Equations
- d
I don't really understand what the 2 integrals (dx and dxdy) for I_x represent. Could I get some explanation here please? Thanks in advance.