Math Amateur
Gold Member
MHB
- 3,920
- 48
I am reading Steve Awodey's book: Category Theory (Second Edition) and am focused on Chapter 2: Abstract Structures ... ...
I need some help in order to fully understand Awodey Example 2.3, Chapter 2 ... ... Awodey Example 2.3, Chapter 2 reads as follows:View attachment 8398
In the Example above Awodey writes the following:
" ... By the UMP of the free monoid $$M(1)$$ ... ... "I am having trouble applying the UMP for the free monoid $$M(A)$$ ... see text below from Awodey pages 18-19 ...
Can someone please help ... in particular I am having trouble identifying the various functions, sets and structures in the UMP on pages 18-19 with the Example ... I can see the set $$A$$ is $$1$$ ... but what is $$i, f, \overline{f}, N $$ in terms of Example 2.3 ... specifically how is Awodey applying the UMP to the Example ... can someone clearly explain the situation ...
Hope that someone can help ...
Peter
=========================================================================================The above post mentions the UMP for free monoids ... so I am providing the text of the same ... ... as follows:
View attachment 8399
View attachment 8400It may also help readers of the above post to have access to the start of Awodey's section on Epis and Monos (up to Example 2.3) ... so I am providing the same ... as follows ...
View attachment 8401
View attachment 8402Hope that helps ...
Peter
I need some help in order to fully understand Awodey Example 2.3, Chapter 2 ... ... Awodey Example 2.3, Chapter 2 reads as follows:View attachment 8398
In the Example above Awodey writes the following:
" ... By the UMP of the free monoid $$M(1)$$ ... ... "I am having trouble applying the UMP for the free monoid $$M(A)$$ ... see text below from Awodey pages 18-19 ...
Can someone please help ... in particular I am having trouble identifying the various functions, sets and structures in the UMP on pages 18-19 with the Example ... I can see the set $$A$$ is $$1$$ ... but what is $$i, f, \overline{f}, N $$ in terms of Example 2.3 ... specifically how is Awodey applying the UMP to the Example ... can someone clearly explain the situation ...
Hope that someone can help ...
Peter
=========================================================================================The above post mentions the UMP for free monoids ... so I am providing the text of the same ... ... as follows:
View attachment 8399
View attachment 8400It may also help readers of the above post to have access to the start of Awodey's section on Epis and Monos (up to Example 2.3) ... so I am providing the same ... as follows ...
View attachment 8401
View attachment 8402Hope that helps ...
Peter
Attachments
-
 Awodey - Example 2.3 ... Page 30 ... .png29.9 KB · Views: 120
Awodey - Example 2.3 ... Page 30 ... .png29.9 KB · Views: 120 -
 Awodey - 1 - UMP for Free Monoids ... PART 1 ... .png5.5 KB · Views: 117
Awodey - 1 - UMP for Free Monoids ... PART 1 ... .png5.5 KB · Views: 117 -
 Awodey - 2 - UMP for Free Monoids ... PART 2 .png11.6 KB · Views: 119
Awodey - 2 - UMP for Free Monoids ... PART 2 .png11.6 KB · Views: 119 -
 Awodey - 1 - Start of Section 2.1, Epis and Monos ... PART 1 .png12.7 KB · Views: 127
Awodey - 1 - Start of Section 2.1, Epis and Monos ... PART 1 .png12.7 KB · Views: 127 -
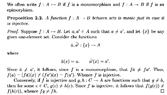 Awodey - 2 - Start of Section 2.1, Epis and Monos ... PART 2 .png16.7 KB · Views: 123
Awodey - 2 - Start of Section 2.1, Epis and Monos ... PART 2 .png16.7 KB · Views: 123