member 731016
- Homework Statement
- Please see below
- Relevant Equations
- Please see below
For this,
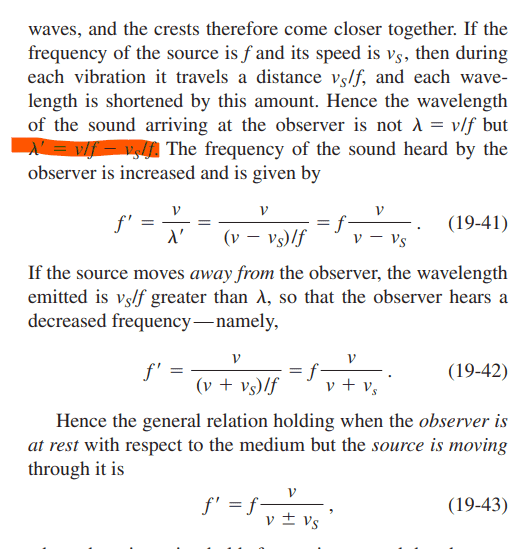
Does someone please know whether they assume for the equation highlighted that ##\frac{v}{f} ≥ \frac{v_S}{f}## since otherwise the wavelength would be negative (which I assume is impossible)?
Many thanks!
Does someone please know whether they assume for the equation highlighted that ##\frac{v}{f} ≥ \frac{v_S}{f}## since otherwise the wavelength would be negative (which I assume is impossible)?
Many thanks!