- #1
Granger
- 168
- 7
Homework Statement
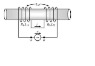
I have the following circuit:
Homework Equations
3. The Attempt at a Solution [/B]
So I thought of applying:
$$K=\frac{|L_M|}{\sqrt{L_1 L_2}}$$
However:
(a) this does not tell me about the sign of $L_M$ (concordant or discordant coupling)
(b) I don't now the self-inductances BUT THEY ARE DIFFERENT.
What should I do with so little numerical info? Any tips?