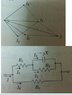
- #1
You are using an out of date browser. It may not display this or other websites correctly.
You should upgrade or use an alternative browser.
You should upgrade or use an alternative browser.
Need explanations about phasor diagram
- Thread starter MissP.25_5
- Start date
-
- Tags
- Diagram Phasor Phasor diagram
In summary: A phasor diagram takes the rotating vectors of the time domain and 'removes' (or ignores) the time dependent component (that would be the ##e^{ωt}## bits). As a result the phasor diagram is a static depiction of the...
Physics news on Phys.org
- #2
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:How do we know that the phase of I1 is faster than I0? And how do we know that I3 is faster than I2?
How do we know that I2 is the slowest of them all??
Okay, a slight misconception there. All of the phasors are assumed to rotate at the same rate. This is tied to the frequency, ω, associated with the driving current.
I think what you are trying to get at is the relative phases of the phasors. Conventionally, for a right-handed coordinate system angles increase from the reference angle in a counterclockwise direction.
- #3
MissP.25_5
- 331
- 0
gneill said:Okay, a slight misconception there. All of the phasors are assumed to rotate at the same rate. This is tied to the frequency, ω, associated with the driving current.
I think what you are trying to get at is the relative phases of the phasors. Conventionally, for a right-handed coordinate system angles increase from the reference angle in a counterclockwise direction.
So, it doesn't matter if I3 and I2 switch places?
- #4
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:So, it doesn't matter if I3 and I2 switch places?
In the diagram I3 is 90° ahead of I2. If they switched places then I2 would be 90° ahead of I3.
- #5
MissP.25_5
- 331
- 0
gneill said:In the diagram I3 is 90° ahead of I2. If they switched places then I2 would be 90° ahead of I3.
How do I know that I3 is ahead of I2?
- #6
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:How do I know that I3 is ahead of I2?
It is counterclockwise from I2. (You look for the smaller of the angles between the two, since by convention phases are taken to lie between -180° and +180°).
- #7
MissP.25_5
- 331
- 0
gneill said:It is counterclockwise from I2. (You look for the smaller of the angles between the two, since by convention phases are taken to lie between -180° and +180°).
I still don't get it :( Why is I3 counterclockwise from I2? Does the circuit diagram indicate anything about the direction?
- #8
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:I still don't get it :( Why is I3 counterclockwise from I2? Does the circuit diagram indicate anything about the direction?
I am going by the provided phasor diagram. Presumably the phasors were determined by an analysis of the circuit.
EDIT: If you consider I2 and I3 in the circuit diagram they are currents through parallel components. That means they share the same potential difference. What do you know about the current phase (with respect to the potential) for those components?
Last edited:
- #9
MissP.25_5
- 331
- 0
gneill said:I am going by the provided phasor diagram. Presumably the phasors were determined by an analysis of the circuit.
EDIT: If you consider I2 and I3 in the circuit diagram they are currents through parallel components. That means they share the same potential difference. What do you know about the current phase (with respect to the potential) for those components?
Oh, you mean I3 in the diagram is above I2, so that's why I3 is ahead of I2 in the phasor diagram?
- #10
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:Oh, you mean I3 in the diagram is above I2, so that's why I3 is ahead of I2 in the phasor diagram?
Consider the two phasors p1 and p2 in the following diagram:
The smaller angle between the two is ##\phi##, so that is the phase difference. p2 is ahead of p1 by phase angle ##\phi##, going counterclockwise (positive direction) from p1 to p2. You could also say that p1 lags p2 by phase angle ##\phi##.
Attachments
- #11
MissP.25_5
- 331
- 0
gneill said:Consider the two phasors p1 and p2 in the following diagram:
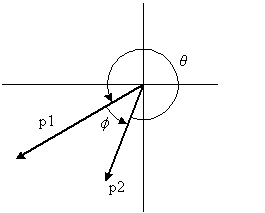
The smaller angle between the two is ##\phi##, so that is the phase difference. p2 is ahead of p1 by phase angle ##\phi##, going counterclockwise (positive direction) from p1 to p2. You could also say that p1 lags p2 by phase angle ##\phi##.
Yes, I got that but what I don't understand is why p2 is ahead of p1?Why not the other way round?
By they way, in the picture I attached, the phasor diagram is the answer. The problem is to find draw the phasor diagram for the circuit (the second picture). But I posted both the problem and answer because I don't understand the answer. I don't know why I2 is ahead of I3. Imagine that the answer isn't there.
Last edited:
- #12
gneill
Mentor
- 20,989
- 2,933
p2 is ahead of p1 because it lies COUNTERCLOCKWISE to p1, moving through the smaller angle between them.MissP.25_5 said:Yes, I got that but what I don't understand is why p2 is ahead of p1?Why not the other way round?
A phasor diagram takes the rotating vectors of the time domain and 'removes' (or ignores) the time dependent component (that would be the ##e^{ωt}## bits). As a result the phasor diagram is a static depiction of the relationships between voltages and currents in a circuit. If the excised bits were left in, you'd need an animated display to depict all the vectors rotating COUNTERCLOCKWISE at a constant rate ω. Phasors COUNTERCLOCKWISE of a given phasor are leading in phase. Those that are CLOCKWISE are lagging.
I3 is ahead because the components carrying the currents share the same potential difference and current LEADS potential by ##\pi/2## radians for a capacitor, but is in step with potential for a resistor. So the capacitor current (I3) must LEAD that of the parallel resistor by ##\pi/2## radians.By they way, in the picture I attached, the phasor diagram is the answer. The problem is to find draw the phasor diagram for the circuit (the second picture). But I posted both the problem and answer because I don't understand the answer. I don't know why I2 is ahead of I3. Imagine that the answer isn't there.
- #13
MissP.25_5
- 331
- 0
gneill said:I3 is ahead because the components carrying the currents share the same potential difference and current LEADS potential by ##\pi/2## radians for a capacitor, but is in step with potential for a resistor. So the capacitor current (I3) must LEAD that of the parallel resistor by ##\pi/2## radians.
Aaaaa...I got it now! This explains a lot. Thank you so much for your patience.
- #14
MissP.25_5
- 331
- 0
gneill said:p2 is ahead of p1 because it lies COUNTERCLOCKWISE to p1, moving through the smaller angle between them.
A phasor diagram takes the rotating vectors of the time domain and 'removes' (or ignores) the time dependent component (that would be the ##e^{ωt}## bits). As a result the phasor diagram is a static depiction of the relationships between voltages and currents in a circuit. If the excised bits were left in, you'd need an animated display to depict all the vectors rotating COUNTERCLOCKWISE at a constant rate ω. Phasors COUNTERCLOCKWISE of a given phasor are leading in phase. Those that are CLOCKWISE are lagging.
I3 is ahead because the components carrying the currents share the same potential difference and current LEADS potential by ##\pi/2## radians for a capacitor, but is in step with potential for a resistor. So the capacitor current (I3) must LEAD that of the parallel resistor by ##\pi/2## radians.
Ok, just to make sure that I really get it, can you check this for me please? Am I drawing it right? Sorry, the picture is a bit blurry but you can still see it, I bet.
Attachments
- #15
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:Ok, just to make sure that I really get it, can you check this for me please? Am I drawing it right? Sorry, the picture is a bit blurry but you can still see it, I bet.
I see that you've taken the current I as the phase reference. Since R1 and L are in series and carry current I, then your diagram is correct for them: V1 is in phase with I, and V2 is advanced by 90° as you've drawn.
V3 and E are a bit trickier. While R2 and C are in parallel as in the previous case in this thread, this time you're looking for voltage phases not current phases, and since the components are in parallel, they must both have the same voltage and phase. While the capacitor current will lead that of R2 by 90°, that doesn't tell us the specific relationship to I, so it doesn't tell us the specific relationship of the voltage phases with respect to I, either. E is also going to have some phase with respect to I, determined by the sum of the other voltage phasors. So the phases for V3 and E are going to depend upon particular component values.
V3's phase will lie somewhere between 0 and -90 degrees with respect to I, but I don't see it being exactly -90°. Depending upon the particular values of the components, and thus where V3 ends up, E could lie above or below I in terms of phase.
I don't suppose you're given component values for this problem?
- #16
MissP.25_5
- 331
- 0
gneill said:I see that you've taken the current I as the phase reference. Since R1 and L are in series and carry current I, then your diagram is correct for them: V1 is in phase with I, and V2 is advanced by 90° as you've drawn.
V3 and E are a bit trickier. While R2 and C are in parallel as in the previous case in this thread, this time you're looking for voltage phases not current phases, and since the components are in parallel, they must both have the same voltage and phase. While the capacitor current will lead that of R2 by 90°, that doesn't tell us the specific relationship to I, so it doesn't tell us the specific relationship of the voltage phases with respect to I, either. E is also going to have some phase with respect to I, determined by the sum of the other voltage phasors. So the phases for V3 and E are going to depend upon particular component values.
V3's phase will lie somewhere between 0 and -90 degrees with respect to I, but I don't see it being exactly -90°. Depending upon the particular values of the components, and thus where V3 ends up, E could lie above or below I in terms of phase.
I don't suppose you're given component values for this problem?
Well, here's the actual question.
In the diagram’s circuit, the effective value of E is 100 [V], angular frequency is 10[rad/s], R1=R2=1 [Ω], L=0.1 [H], C=0.1 [F].
1) Draw the phasor diagram (vector diagram) of the relation between E, V1, V2, V3 and I.
2) Find the effective power (power consumption) of the entire circuit.
I got the answer for the second question, P=6000W.
- #17
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:Well, here's the actual question.
In the diagram’s circuit, the effective value of E is 100 [V], angular frequency is 10[rad/s], R1=R2=1 [Ω], L=0.1 [H], C=0.1 [F].
1) Draw the phasor diagram (vector diagram) of the relation between E, V1, V2, V3 and I.
2) Find the effective power (power consumption) of the entire circuit.
I got the answer for the second question, P=6000W.
So it looks like E should provide your reference phase (that is, it is assumed to have a phase of zero). I'd look at solving for the total impedance first, then find the total current I = E/Z. Then you can determine V1, V2, and V3 pretty easily. Do all this using complex values and you'll be able to extract the magnitudes and phases.
Your effective power value looks fine.
- #18
MissP.25_5
- 331
- 0
gneill said:So it looks like E should provide your reference phase (that is, it is assumed to have a phase of zero). I'd look at solving for the total impedance first, then find the total current I = E/Z. Then you can determine V1, V2, and V3 pretty easily. Do all this using complex values and you'll be able to extract the magnitudes and phases.
Your effective power value looks fine.
Oh, I forgot something. In the question, it actually says to draw the phasor of the relation between all those vectors. So I assume that when it says "relation", we don't need to draw it very specifically, do we?
- #19
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:Oh, I forgot something. In the question, it actually says to draw the phasor of the relation between all those vectors. So I assume that when it says "relation", we don't need to draw it very specifically, do we?
Right, you don't need to choose a particular orientation. However, it's convenient to pick one of the phasors as a reference to work from . The supply voltage E looks to be convenient since its RMS magnitude is given, and in practice the source is generally the reference anyways.
- #20
MissP.25_5
- 331
- 0
gneill said:Right, you don't need to choose a particular orientation. However, it's convenient to pick one of the phasors as a reference to work from . The supply voltage E looks to be convenient since its RMS magnitude is given, and in practice the source is generally the reference anyways.
So is my drawing wrong?
- #21
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:So is my drawing wrong?
I would reduce the angle between V3 and I. V2 and V3 are definitely not co-linear.
- #22
MissP.25_5
- 331
- 0
gneill said:So it looks like E should provide your reference phase (that is, it is assumed to have a phase of zero). I'd look at solving for the total impedance first, then find the total current I = E/Z. Then you can determine V1, V2, and V3 pretty easily. Do all this using complex values and you'll be able to extract the magnitudes and phases.
Your effective power value looks fine.
Uhmm...how do you determine whether or not to take E as the reference?
- #23
gneill
Mentor
- 20,989
- 2,933
As I stated previously (post #19), the power source for a circuit traditionally serves as the reference. It's not a rigid rule, so you may choose another current or voltage if you wish; it'll only alter the orientation of the diagram.MissP.25_5 said:Uhmm...how do you determine whether or not to take E as the reference?
- #24
MissP.25_5
- 331
- 0
gneill said:As I stated previously (post #19), the power source for a circuit traditionally serves as the reference. It's not a rigid rule, so you may choose another current or voltage if you wish; it'll only alter the orientation of the diagram.
Oh, I get it now. I fixed my drawing. Does this seem right?
Attachments
- #25
gneill
Mentor
- 20,989
- 2,933
MissP.25_5 said:Oh, I get it now. I fixed my drawing. Does this seem right?
Yes, that looks fine.
- #26
MissP.25_5
- 331
- 0
gneill said:Yes, that looks fine.
THANK YOU SO MUCH!I posted a new thread, just to make sure I got it right. Can you take a look, please? After exam, I won't bother you as much anymore, haha.
FAQ: Need explanations about phasor diagram
1. What is a phasor diagram?
A phasor diagram is a graphical representation of a complex sinusoidal wave. It is used in electrical engineering to visualize the amplitude and phase relationship between two or more sinusoidal signals.
2. How is a phasor diagram helpful in circuit analysis?
A phasor diagram is helpful in circuit analysis because it allows us to simplify complex sinusoidal signals into a single vector. This makes it easier to analyze the behavior of a circuit and determine the overall voltage and current at any given point.
3. What are the components of a phasor diagram?
The components of a phasor diagram include the magnitude and phase angle of the sinusoidal signals, represented by the length and angle of the vector respectively. These vectors can also be combined using vector addition to show the resulting waveform.
4. How is the phase difference between two signals represented in a phasor diagram?
The phase difference between two signals is represented by the angle between their respective phasors. A positive angle indicates a leading phase, while a negative angle indicates a lagging phase. A phase difference of 90 degrees would result in perpendicular phasors.
5. Can a phasor diagram be used for non-sinusoidal signals?
While a phasor diagram is primarily used for sinusoidal signals, it can also be used for non-sinusoidal signals by breaking them down into their harmonic components. However, the resulting phasor diagram may not accurately represent the original waveform.
Similar threads
Engineering
AC Circuit Phasors: Find I1, I2, I3
- Replies
- 26
- Views
- 2K
- Replies
- 5
- Views
- 2K
- Replies
- 4
- Views
- 2K
- Replies
- 1
- Views
- 2K
- Replies
- 3
- Views
- 2K
- Replies
- 1
- Views
- 2K
- Replies
- 1
- Views
- 2K
- Replies
- 6
- Views
- 4K
- Replies
- 14
- Views
- 2K
- Replies
- 20
- Views
- 3K
Share: