Net charge contained by a cube in a region with a non-uniform E field

[mhrob24]

Homework Statement

The figure shows a closed Gaussian surface in the shape of a cube with edge length of 2m. It lies in a region where the non-uniform electric field is given by: E = (3.00x+4.00)i + 6.00j +7.00k N/C, with "x" in meters. What is the net charge contained by the cube?

Relevant Equations

Flux = E * A = Q(enclosed)/ε0

I'm having a little trouble understanding how to go about solving this problem. I was in class Tuesday and the hint I got from the T.A. running my discussion section was that : "because the electric field is only non-uniform along the x axis, the electric field will both enter(negative flux) and exit(positive flux)the faces along the y and z axis the same, so there will be no net electric flux going through those 4 faces"

Now, taking what he says to be the truth, that would mean to solve this problem, I would simply use : E * A= Q(enclosed)/ε0 , plug in the value for the electric field along the x axis, plug in the value for "x", and solve for Q(enclosed) which would look like: Q(enclosed) = 3.00(2m)+4.00 * A * ε0

However, something I read in my textbook makes me feel like the hint I was given by the T.A. wasn't exactly correct. In my textbook (when talking about electric flux from a uniform electric field through a cube ), it reads:

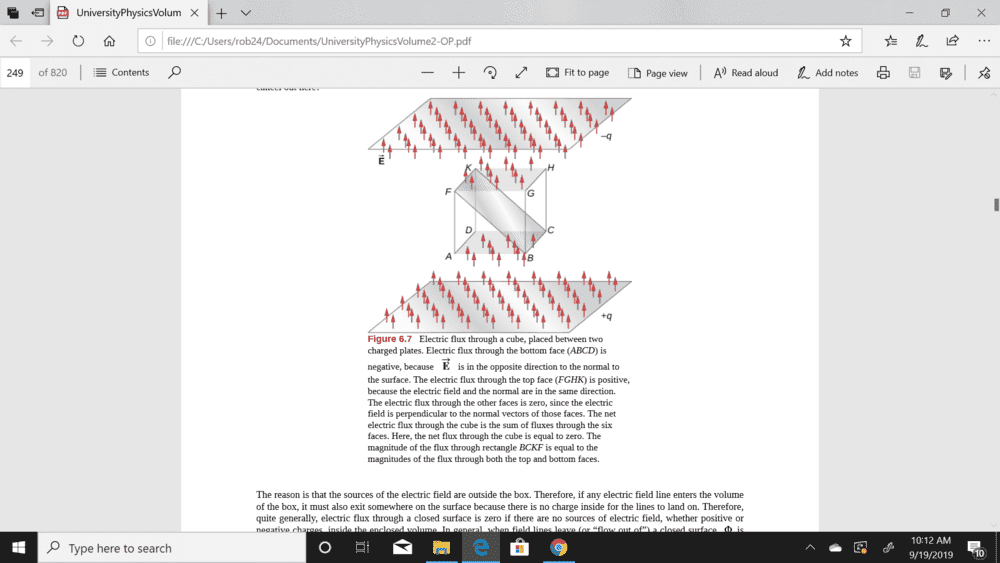
"The sources of the electric field are outside of the cube. Therefore, if any electric field line enters the volume of the cube, it must also exit somewhere on the surface because there is no charge for the field lines to land on. This means that generally, electric flux through a closed surface is zero if there are no charges (positive or negative) inside the cube."

Now, if this is the case, then what I was told can't be true because the question I am asked to solve is "what is the net CHARGE CONTAINED BY THE CUBE?", meaning that the cube does indeed contain a net charge. So according to my textbook, the net flux through the faces along the y and z axis can't just be zero because there is a charge for those field lines to land on. So now I am just really confused on what to do. Can someone get me in the right direction so I can solve this problem? Thank you!

PS: Here is the diagram I was given for this problem along with the quote I gave from my textbook:

 

[Orodruin]

However, something I read in my textbook makes me feel like the hint I was given by the T.A. wasn't exactly correct. In my textbook (when talking about electric flux from a uniform electric field through a cube ),

This is talking about a uniform field. Your field is not uniform.

Edit: So, put logically, your textbook is saying field line in means field line out because there are no charges to "land on". This does not mean that charges to "land on" means that a particular field line must "land on" a charge, just that some field lines do.

 

[mhrob24]

I'm still confused for 2 reasons:

1. the field along the y and z axis in my problem IS uniform (from what I was told by my T.A.) because it doesn't depend on where you are along the y or z axis; whereas the field along the x-axis is not uniform because it depends on the distance "x".

2. From what you're saying, that would mean that not all electric field lines will land on the charge inside the cube, only some will. If that is the case, then what I was told was indeed incorrect because that means that the flux going through the faces along the y and z axis won't just cancel out because SOME of the field lines will land on the net charge, thus the field won't exit and enter with the same value. I was told by my T.A. the flux going in and out of these faces will cancel...

 

[haruspex]

Q(enclosed) = 3.00(2m)+4.00 * A * ε0

That's the flux out through one end. You need to subtract what came in at the other.

From what you're saying, that would mean that not all electric field lines will land on the charge inside the cube, only some will.

Yes, but that does not mean the fluxes through the faces parallel to the x-axis do not cancel. It can just mean that the fluxes through the faces normal to the x-axis do not cancel.