paulimerci
- 287
- 47
- Homework Statement
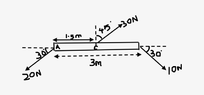
- What is the net torque about an axis through point A?
- Relevant Equations
- Torque = F X d X sin theta
The net torque about an axis through point A is given by,
If I take the axis of rotation perpendicular to the paper and the solution I arrive would be the following below
Net torque = 30 cos45 x 1.5 - 10 cos30X 3
= 5.829Nm ( counterclockwise)
But the book gives an answer like this,
Net torque = 30 cos 45x1.5 - 10 x sin30 x 3 = 16.8Nm.What did I miss? It looks the horizontal component is perpendicular to the axis of rotation.
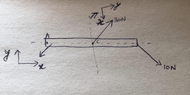
If I take the axis of rotation perpendicular to the paper and the solution I arrive would be the following below
Net torque = 30 cos45 x 1.5 - 10 cos30X 3
= 5.829Nm ( counterclockwise)
But the book gives an answer like this,
Net torque = 30 cos 45x1.5 - 10 x sin30 x 3 = 16.8Nm.What did I miss? It looks the horizontal component is perpendicular to the axis of rotation.