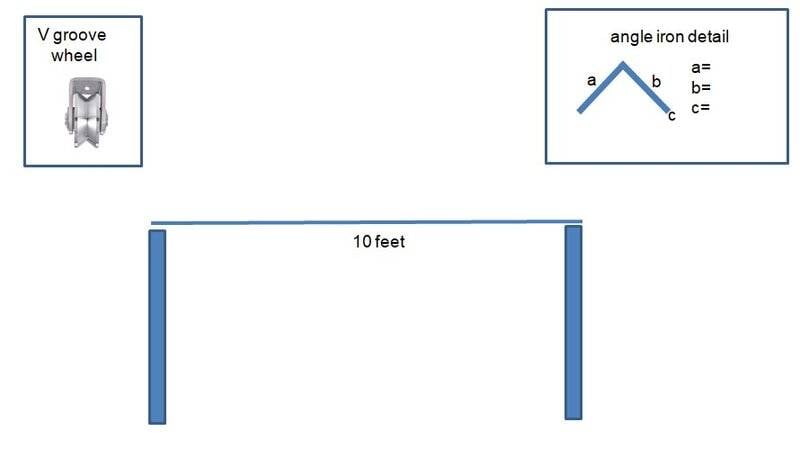
peabody1998 said:
I have simplified the question further to get a rough idea of what load aluminum vs steel angle can handle.
For example: Given 2"x2"x0.25" angle oriented vertically like '^' supported 10 ft apart with a point weight of 200lbs midway, what is the deflection with aluminum? steel?
You haven't simplified the question, you changed it. You asked about "the load that could be safely carried by" and now you are asking about the deflection.
The equations to calculate the maximum deflection for a certain beam under a certain load can be found
here - together with a calculator. For the basic case you are asking about:
$$\delta_{max} = \frac{PL^3}{48EI}$$
To calculate the second moment of area ##I##, you can find good info - and a calculator - for an angle beam
here.
You can find the typical values for Young's modulus ##E##
here, i.e. 68 GPa for aluminum and 200 GPa for steel A36.
As for the "safe load" you need to find the maximum stress within your beam. You have the equation
here, namely:
$$\sigma = \frac{Mc}{I}$$
We already know ##I## and ##c## is just the longest distance, perpendicularly, from the neutral axis.
For the maximum moment ##M## found on the beam, you can use
this calculator and get the maximum value on the moment diagram. For the simple case proposed (a beam of length ##L## with a load ##P## at its center), it is:
$$M_{max} = \frac{PL}{4}$$
For more info on how it is done, find it
here (fun stuff!).
The beam will permanently deform once the stress ##\sigma## gets larger than the yield strength of the material. Some typical values can be found
here, i.e. 250 MPa for steel A36 and 400 MPa for Aluminum alloy 2014-T6. These numbers can vary widely from different alloys, even different manufacturers.
Then you have to consider the
safety factor, which is the yield stress divided by the working stress we calculated. If you are using the equations and approximate numbers I presented here - together with the very simple description of your problem and the obvious lack of knowledge you have about what needs to be done - you probably need a safety factor of at least 10 to be really on the safe side.
And this is why nobody on this forum will give you a straight answer - especially the "safe load" - to this seemingly simple but, oh!, very complex problem.