Newton's law problem: Pushing 2 stacked blocks on a horizontal table

[JMAMA]

Homework Statement

solve for acceleration of block A

Relevant Equations

f = ma

Would anyone be able to help with this Newtons law problem
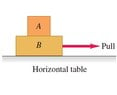
Block A rests on top of block B as shown in (Figure 1). The table is frictionless but there is friction (a horizontal force) between blocks A and B. Block B has mass 6.00 kg and block A has mass 2.00 kg. If the horizontal pull applied to block B equals 12.0 N, then block B has an acceleration of 1.80 m/s2. What is the acceleration of block A

So my understanding is that from F = ma we can determine for block B that F = (6 kg)(1.80 ms2) = 10.80 N we can then say that block A is experiencing the same force yielding F - F(friction) = m a or 10.8 N - (2.0kg)(g)u = (2kg)(a). However i do not know how to solve for acceleration of A without the coefficient of friction

 

[kuruman]

According to our rules, to receive help, you need to show some credible effort towards answering the question. How about telling us what you do know and how you would approach this problem?

Please read, understand and follow our homework guidelines, especially item 4, here
https://www.physicsforums.com/threads/homework-help-guidelines-for-students-and-helpers.686781/

 

[JMAMA]

So my understanding is that from F = ma we can determine for block B that F = (6 kg)(1.80 ms2) = 10.80 N we can then say that block A is experiencing the same force yielding F - F(friction) = m a or 10.8 N - (2.0kg)(g)u = (2kg)(a). However i do not know how to solve for acceleration of A without the coefficient of friction

 

[kuruman]

It would help if you posted the figure that goes with this problem. Use the link button "Attach files" on the lower left.

 

[JMAMA]

It would help if you posted the figure that goes with this problem. Use the link button "Attach files" on the lower left.

attached in edit

 

[kuruman]

Thanks. Now consider this. How many forces act on the top block and what object(s) exert(s) them on it?

 

[JMAMA]

Thanks. Now consider this. How many forces act on the top block and what object(s) exert(s) them on it?

there is the gravitational force, the applied force, and the frictional force the applied force is applied by the bottom block

 

[Lnewqban]

So my understanding is that from F = ma we can determine for block B that F = (6 kg)(1.80 ms2) = 10.80 N we can then say that block A is experiencing the same force yielding F - F(friction) = m a or 10.8 N - (2.0kg)(g)u = (2kg)(a). However i do not know how to solve for acceleration of A without the coefficient of friction

Welcome, @JMAMA !

You don’t need to calculate that force of friction in this case.
That pulling force is able to accelerate a mass of 6.66 kg at the given rate.
Therefore, it is simultaneously accelerating block B at that rate, and block A at a different rate.

 

[Cutter Ketch]

Draw the free body diagram for B. The method for solving the problem should then be apparent.

 

[JMAMA]

Welcome, @JMAMA !

You don’t need to calculate that force of friction in this case.
That pulling force is able to accelerate a mass of 6.66 kg at the given rate.
Therefore, it is simultaneously accelerating block B at that rate, and block A at a different rate.

what would the equation be for that the 0 friction is throwing me off. would it just be 6 m/s2 from 12N = 2kg a??

 

[JMAMA]

Draw the free body diagram for B. The method for solving the problem should then be apparent.

 

[Cutter Ketch]

Well, sort of. A) What is that force to the left? Where does it come from. B) it’s not F=ma as you indicated for relevant equations, it’s
$\sum F$ = ma so what should you do with that free body diagram?

 

[erobz]

Outline:

1) Draw a FBD for block A

2) Write Newtons Second Law for block A

3) Draw FBD for Block B

4) Write Newtons Second Law for block B

5) Combine the two equations, solve for the acceleration of block $A$

Where you might get stuck...the frictional force acting between blocks A and B. If it necessarily acts on block $A$ in a certain direction, in which way does it act on block $B$?

 

[Lnewqban]

what would the equation be for that the 0 friction is throwing me off. would it just be 6 m/s2 from 12N = 2kg a??

Can you visualize block A accelerating at a lower rate than block B and falling behind?

If no friction existed between A and B, block A would not move respect to the ground, and our force of 12.0 N would induce a greater acceleration than 1.8 m/s² to block B 9only one experiencing that force then).

If blocks A and B were solidly linked together, both blocks would move respect to the ground with identical acceleration, and our force of 12.0 N would induce a lower acceleration than 1.8 m/s² to the A-B block system of mass 8.0 kg.