Newton's Law's Problem (Contact Force)

[certainlynot5]

Basically 3 boxes, somehting like this;

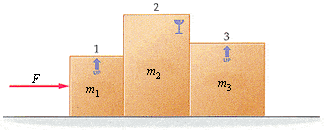
Masses are as followed: M1= 28 kg, M2= 18.7 kg, M3= 28.7 kg. The applied force on the box is 644 N

Part A) What is the magnitude of the contact force between M1 and M2
Part B) What is the magnitude of the contact force between M2 and M3


To find the contace force between M1 and M2, i used this equation; 644/(28 + 18.7) for Part A, and 644/(18.7 + 28.7) for Part B. The anwers are; Part A, 13.79, and for Part B, 13.58.

Is this the correct method? If not, what can be done to achieve the correct answer?

 

[PhanthomJay]

Start by finding the acceleration of the total system of three blocks. Then take each block apart in a free body diagram, identify all forces acting on each block, and apply Newton's laws.

 

[certainlynot5]

and to find acceleration, i would use the formula, F/mg which is 644/28, 644/18.8, 644/28.7?

 

[PhanthomJay]

and to find acceleration, i would use the formula, F/mg which is 644/28, 644/18.8, 644/28.7?

No, each block is in contact with each other, so they must each accelertae at the same rate. What is the acceleration of the total system of the 3 blocks together (as if they were one big mass)?

 

[certainlynot5]

the the entire mass is 75.4

 

[PhanthomJay]

the the entire mass is 75.4

Yes, in kg. So what's the acceleration of the entire mass?