Woopa
- 20
- 4
I am having difficulty understanding the information below. In the second line it states that F=0 as there is no external force on the system. However it later calculates acceleration of the rocket.
How can Force=0 if there is acceleration? (This is the first time I have encountered the product rule so this may be part of my misunderstanding)
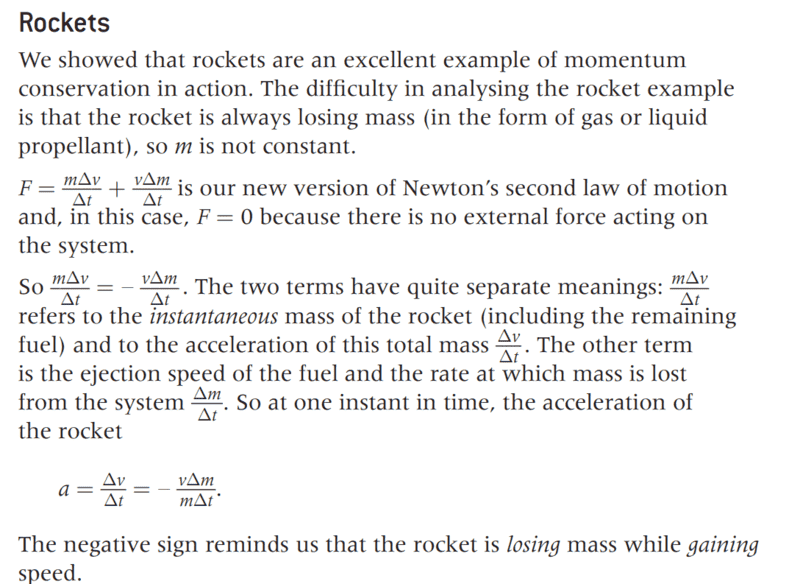
How can Force=0 if there is acceleration? (This is the first time I have encountered the product rule so this may be part of my misunderstanding)