Non-inertial observer inside train

In summary, the observer inside the train cannot directly measure the acceleration of the train. They can only measure the deflection in a spring scale and use that information to calculate the acceleration. This is because they are unable to see the increasing velocity of the train or the boxcar. Additionally, in the perspective of the observer inside the train, the fictitious force acting on the mass until the string becomes taut has a very real effect. However, from an external, inertial frame of reference, this force is considered fictitious because it depends on the mass of the object being acted on, which is not a characteristic of real forces.
  • #1
member 731016
Homework Statement
Pls see below
Relevant Equations
Pls see below
For part (c) of this problem,
1676851122866.png

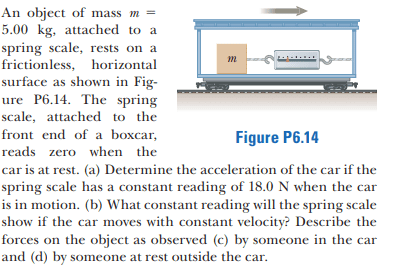
The solution is,
1676851157115.png

However, how did they know that the fictitious force that acts on the mass until the string become taut (T = Ma from as observed by an observer at rest outside) has a magnitude of Ma (assuming that the observer has no communication with the outside observer)

Many thanks!

Many thanks!
 
Physics news on Phys.org
  • #2
Callumnc1 said:
...
However, how did they know that the fictitious force that acts on the mass until the string become taut (T = Ma from as observed by an observer at rest outside) has a magnitude of Ma (assuming that the observer has no communication with the outside observer)
Someone in the car can only see how much deflection occurs in the spring scale.
That person is unable to see the increasing velocity of the object or the boxcar.
Therefore, a direct measurement of the acceleration can't be made by that person.
 
  • Like
Likes member 731016
  • #3
Callumnc1 said:
how did they know that the fictitious force that acts on the mass until the string become taut (T = Ma from as observed by an observer at rest outside) has a magnitude of Ma (assuming that the observer has no communication with the outside observer)
The observer in the car has to have some way of figuring out the acceleration of the car. Note that they have no way to distinguish a literal acceleration from a constant horizontal gravitational attraction. This is part of the reasoning behind Einstein's GR theory.
 
  • Like
Likes member 731016
  • #4
Thank you for your replies @Lnewqban and @haruspex !

I guess we could go as far as saying then that there is no way to tell from an non-inertial perspective inside the carriage that there is a fictitious force acting at all. From their perspective it is a very real force, correct?

Many thanks!
 
  • Skeptical
Likes PeroK
  • #5
Callumnc1 said:
Thank you for your replies @Lnewqban and @haruspex !

I guess we could go as far as saying then that there is no way to tell from an non-inertial perspective inside the carriage that there is a fictitious force acting at all. From their perspective it is a very real force, correct?

Many thanks!
In GR, gravity is considered a fictitious force because, like acceleration of the frame, it depends on the mass of the object being acted on. Real forces should not depend on that.
 
  • Like
Likes member 731016
  • #6
Callumnc1 said:
Thank you for your replies @Lnewqban and @haruspex !

I guess we could go as far as saying then that there is no way to tell from an non-inertial perspective inside the carriage that there is a fictitious force acting at all. From their perspective it is a very real force, correct?

Many thanks!
Yes. Furthermore, the person inside the train will be under the impression that the "floor" on which the mass rests is an inclined plane. That's because a plumb bob hanging from the ceiling defines the local direction of "down". When viewed from the inertial frame of the ground, the plumb bob will not be perpendicular to the floor, but will be slanted back. Thus, the "down" direction for the person in the car is not perpendicular to the floor. Also, the body of the person must be aligned with the plumb bob and lean into the "incline" to avoid being toppled over. The effective weight of the train rider is the vector sum ##m\mathbf{g}+m(-\mathbf{a})##.
 
  • Like
Likes Lnewqban and member 731016
  • #7
haruspex said:
In GR, gravity is considered a fictitious force because, like acceleration of the frame, it depends on the mass of the object being acted on. Real forces should not depend on that.
Thank you for your help @haruspex !
 
  • #8
kuruman said:
Yes. Furthermore, the person inside the train will be under the impression that the "floor" on which the mass rests is an inclined plane. That's because a plumb bob hanging from the ceiling defines the local direction of "down". When viewed from the inertial frame of the ground, the plumb bob will not be perpendicular to the floor, but will be slanted back. Thus, the "down" direction for the person in the car is not perpendicular to the floor. Also, the body of the person must be aligned with the plumb bob and lean into the "incline" to avoid being toppled over. The effective weight of the train rider is the vector sum ##m\mathbf{g}+m(-\mathbf{a})##.
Thank you for you reply @kuruman !

That is very interesting what you mention about the person having to a higher effective weight. But I guess if the acceleration is purely horizontal in the train's case the normal force from the floor dose not have to increase, correct?

Many thanks!
 
  • #9
Callumnc1 said:
Thank you for you reply @kuruman !

That is very interesting what you mention about the person having to a higher effective weight. But I guess if the acceleration is purely horizontal in the train's case the normal force from the floor dose not have to increase, correct?

Many thanks!
The normal force from the floor does not change, but the person now experiences 'gravity' as being at an angle to the floor, and increased in magnitude.
 
  • Like
Likes member 731016
  • #10
haruspex said:
The normal force from the floor does not change, but the person now experiences 'gravity' as being at an angle to the floor, and increased in magnitude.
Thank you for that @haruspex !
 
  • #11
Callumnc1 said:
Thank you for your replies @Lnewqban and @haruspex !

I guess we could go as far as saying then that there is no way to tell from an non-inertial perspective inside the carriage that there is a fictitious force acting at all. From their perspective it is a very real force, correct?

Many thanks!
Thank you for your reaction @PeroK !

Out of curiosity, what makes you skeptical?

Many thanks!
 
  • #12
Callumnc1 said:
Thank you for your reaction @PeroK !

Out of curiosity, what makes you skeptical?

Many thanks!
You confused force with acceleration. If you are in a car that accelerates forward, then the force you experience is in the forward direction. If something isn't accelerating with the car, then in the reference frame of the car, it is accelerating in the opposite direction. You then must add a fictitious force to explain the relative acceleration in the non-inertial frame.
 
  • Like
Likes Lnewqban and member 731016
  • #13
PeroK said:
You confused force with acceleration. If you are in a car that accelerates forward, then the force you experience is in the forward direction. If something isn't accelerating with the car, then in the reference frame of the car, it is accelerating in the opposite direction. You then must add a fictitious force to explain the relative acceleration in the non-inertial frame.
Thank you help @PeroK !
 

FAQ: Non-inertial observer inside train

What is a non-inertial observer inside a train?

A non-inertial observer inside a train is someone who is experiencing acceleration or deceleration while inside the train. This means that the observer is in a reference frame that is not moving at a constant velocity, and they will feel forces due to the train's acceleration or deceleration.

How does the motion of the train affect a non-inertial observer?

The motion of the train affects a non-inertial observer by causing them to experience fictitious forces. For example, if the train accelerates forward, the observer will feel a force pushing them backward. These forces are not due to any physical interaction but are a result of the acceleration of the reference frame itself.

What are fictitious forces, and how do they apply to a non-inertial observer inside a train?

Fictitious forces, also known as pseudo-forces, are apparent forces that a non-inertial observer experiences due to the acceleration of their reference frame. Inside a train, these could include forces that push the observer backward when the train accelerates forward, or sideways when the train takes a turn. These forces are not caused by physical interactions but are perceived due to the changing velocity of the train.

How can we describe the motion of objects relative to a non-inertial observer inside a train?

To describe the motion of objects relative to a non-inertial observer inside a train, we must account for the fictitious forces acting on those objects. For example, if an object is placed on a table inside an accelerating train, it will appear to slide backward relative to the train. This motion can be explained by adding a fictitious force opposite to the direction of the train's acceleration acting on the object.

Can the laws of physics be applied in the same way for a non-inertial observer inside a train?

The laws of physics can still be applied for a non-inertial observer inside a train, but they need to be modified to include fictitious forces. Newton's laws of motion, for instance, must be adjusted to account for these additional forces to accurately describe the motion of objects from the perspective of the non-inertial observer.

Similar threads

Pendulum hung from the ceiling of a train
Replies
18
Views
3K
Problem about non-inertial reference frame
Replies
8
Views
567
Frame of reference (non inertial/inertial) in an Atwood Mach
Replies
13
Views
2K
Non-inertial Frames of Reference
2
Replies
44
Views
4K
Balloon in Train: Angles of A, B, and C Explained
Replies
21
Views
3K
How to avoid the use of a pseudo force
Replies
15
Views
2K
De Broglie wavelength of a train
Replies
1
Views
1K
Understanding Inertial and Non-Inertial Reference Frames
Replies
5
Views
6K
Atwood's with a cylinder - Rotational Motion
Replies
12
Views
347
Acceleration and velocity: Car and train problem
Replies
3
Views
4K

Hot Threads

Recent Insights

Back
Top