- #1
JD_PM
- 1,131
- 158
I am studying the cavitation theory proposed by https://www.researchgate.net/profile/Guenter-Schnerr-Professor-Dr-Inghabil/publication/296196752_Physical_and_Numerical_Modeling_of_Unsteady_Cavitation_Dynamics/links/56f6b62308ae81582bf2f940/Physical-and-Numerical-Modeling-of-Unsteady-Cavitation-Dynamics.pdf (SS) and realized it assumes isothermal conditions within liquid-gas homogeneous mixture as well as incompressible flow. This is alright for, say, water. However, for cryogenic fluids (such as liquid nitrogen) this assumption is no longer valid.
I want to incorporate non-isothermal phenomena in the SS cavitation theory. This is how I am approaching it
To describe the fluid mechanics, we need to work with1) The continuity equation
\begin{equation}
\frac{\partial \rho}{\partial t} + \nabla \cdot (\rho v) = 0
\end{equation}
Mass conservation holds anyway once non-isothermal effects are incorporated.2) Momentum equation
\begin{equation}
\frac{\partial}{\partial t}(\rho \vec v) + \nabla \cdot (\rho \vec v \vec v) = -\nabla p + \mu \nabla^2 \vec v + \rho \vec g + \vec S
\end{equation}
Where ##\mu## is the viscosity and ##\vec S## is the surface tension force due to the interface interaction between phases.
Momentum conservation is independent of non-isothermal effects.
Given that the SS model deals with incompressible, isothermal flow the energy equation is not required. However, we want to incorporate non-isothermal effects. To do so, we need to include it
\begin{equation}
\frac{\partial}{\partial t}(E \rho) + \nabla \cdot (\rho E \vec v) = \rho \dot q - \nabla \cdot (p \vec v ) + \rho (\vec f \cdot \vec v) + \text{viscous terms}
\end{equation}
Where ##E## is the total energy ##E := u + \frac{V^2}{2}## and ##u## the internal energy.
Where ##\dot q## is the rate of heat and ##\vec f## refers to external forces.
Given the liquid-gas mixture, the following density and viscosity equations are set to be
$$\rho = \alpha_l \rho_l + (1 - \alpha_l ) \rho_l, \ \ \ \ \mu = \alpha_l \mu_l + (1 - \alpha_l ) \mu_l$$
Where ##\alpha_l, \alpha_v## are the liquid and vapor volume fraction respectively and ##\alpha_l = 1## means there is all liquid and ##\alpha_l = 0## all vapor. It is common practice to use ##\alpha_l + \alpha_v = 1## to eliminate ##\alpha_v##.
A new variable, ##\alpha_l##, has been introduced. Hence, a new equation must be included. The liquid-vapor mass transfer (evaporation and condensation) is governed by the vapor transport equation:
$$\frac{\partial}{\partial t} (\alpha_l \rho) + \nabla \cdot (\alpha_l \rho \vec v) = \dot m^{+} + \dot m^{-}$$
Where ##\dot m^{+}, \dot m^{-}## represent, respectively, the evaporation and condensation mass transfer rates which model the mechanism of cavitation.
Note that for incompressible flow (constant density) the transport equation takes the simple form
$$\frac{\partial}{\partial t} (\alpha_l) + \nabla \cdot (\alpha_l \vec v) = \frac{\dot m^{+} + \dot m^{-}}{\rho}$$
The above are the fundamental equations. Let's now turn to the SS model
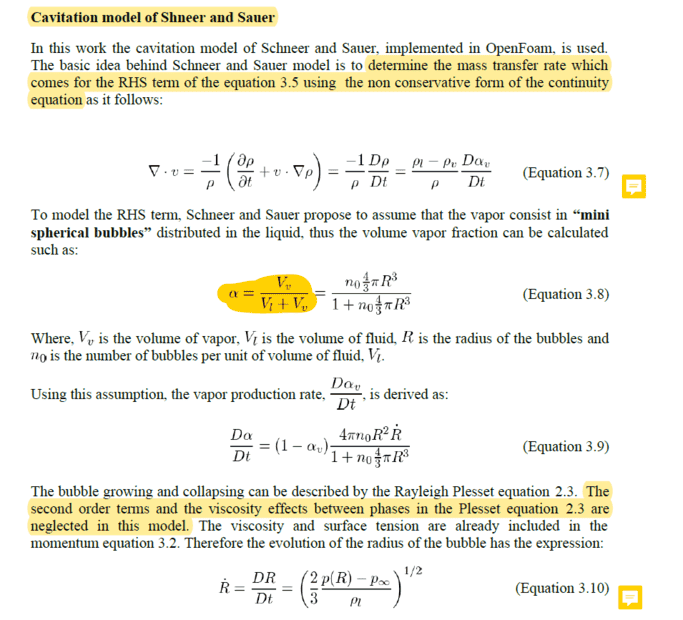
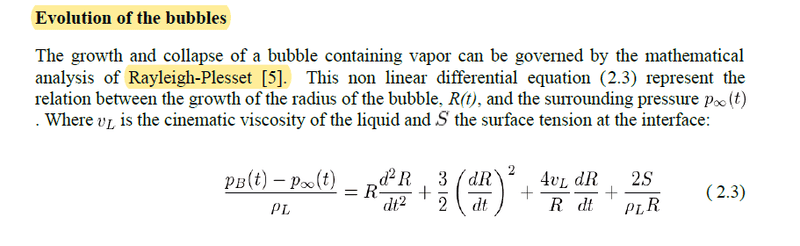
Where equation 3.5 above is the transport equation.
Questions
Thank you!
I want to incorporate non-isothermal phenomena in the SS cavitation theory. This is how I am approaching it
To describe the fluid mechanics, we need to work with1) The continuity equation
\begin{equation}
\frac{\partial \rho}{\partial t} + \nabla \cdot (\rho v) = 0
\end{equation}
Mass conservation holds anyway once non-isothermal effects are incorporated.2) Momentum equation
\begin{equation}
\frac{\partial}{\partial t}(\rho \vec v) + \nabla \cdot (\rho \vec v \vec v) = -\nabla p + \mu \nabla^2 \vec v + \rho \vec g + \vec S
\end{equation}
Where ##\mu## is the viscosity and ##\vec S## is the surface tension force due to the interface interaction between phases.
Momentum conservation is independent of non-isothermal effects.
Given that the SS model deals with incompressible, isothermal flow the energy equation is not required. However, we want to incorporate non-isothermal effects. To do so, we need to include it
\begin{equation}
\frac{\partial}{\partial t}(E \rho) + \nabla \cdot (\rho E \vec v) = \rho \dot q - \nabla \cdot (p \vec v ) + \rho (\vec f \cdot \vec v) + \text{viscous terms}
\end{equation}
Where ##E## is the total energy ##E := u + \frac{V^2}{2}## and ##u## the internal energy.
Where ##\dot q## is the rate of heat and ##\vec f## refers to external forces.
Given the liquid-gas mixture, the following density and viscosity equations are set to be
$$\rho = \alpha_l \rho_l + (1 - \alpha_l ) \rho_l, \ \ \ \ \mu = \alpha_l \mu_l + (1 - \alpha_l ) \mu_l$$
Where ##\alpha_l, \alpha_v## are the liquid and vapor volume fraction respectively and ##\alpha_l = 1## means there is all liquid and ##\alpha_l = 0## all vapor. It is common practice to use ##\alpha_l + \alpha_v = 1## to eliminate ##\alpha_v##.
A new variable, ##\alpha_l##, has been introduced. Hence, a new equation must be included. The liquid-vapor mass transfer (evaporation and condensation) is governed by the vapor transport equation:
$$\frac{\partial}{\partial t} (\alpha_l \rho) + \nabla \cdot (\alpha_l \rho \vec v) = \dot m^{+} + \dot m^{-}$$
Where ##\dot m^{+}, \dot m^{-}## represent, respectively, the evaporation and condensation mass transfer rates which model the mechanism of cavitation.
Note that for incompressible flow (constant density) the transport equation takes the simple form
$$\frac{\partial}{\partial t} (\alpha_l) + \nabla \cdot (\alpha_l \vec v) = \frac{\dot m^{+} + \dot m^{-}}{\rho}$$
The above are the fundamental equations. Let's now turn to the SS model
Where equation 3.5 above is the transport equation.
Questions
- Does including the energy equation simply incorporate non-isothermal conditions to the LN2 mixture?
- Regarding incorporating non-isothermal conditions to the SS model: I see that equation 3.7 would take a more complicated final form, given that the density is no longer constant. Is that the only change?
Thank you!