Non-linear isotropic dielectric capacitor

[milkism]

Homework Statement

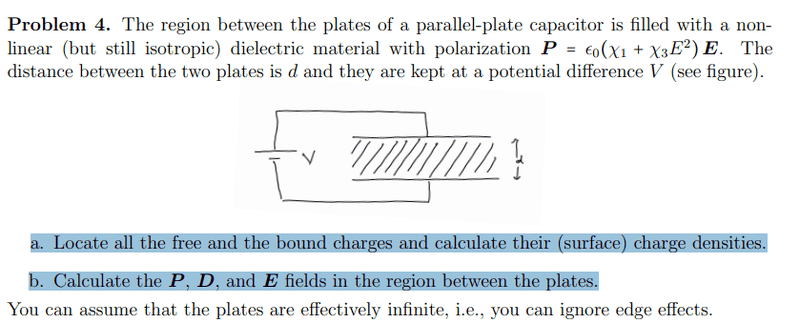
a. Identify the location of all the free and bound charges present in the region between the plates of the parallel-plate capacitor, and determine the surface charge densities associated with them.

b. Determine the values of the polarization (P), electric displacement (D), and electric field (E) in the region between the plates.

Relevant Equations

See solution.

Question:

Solution first part:

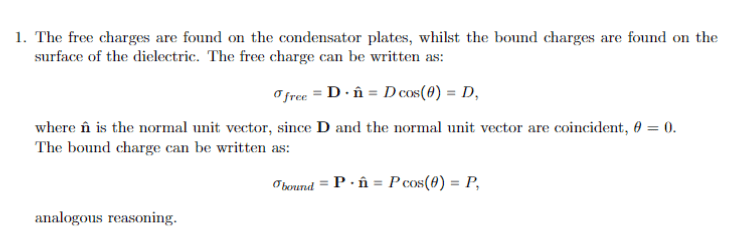
Have I done it right?

I don't know how to begin with second part since the dielectric is non-lineair, and most formulas like $$
D=\epsilon E$$ and $$P= \epsilon_0 \xhi_e E$$, only apply for lineair dielectrics. What to do?

 

[pasmith]

I don't know how to begin with second part since the dielectric is non-lineair, and most formulas like $$
D=\epsilon E$$ and $$P= \epsilon_0 \chi_e E$$, only apply for lineair dielectrics. What to do?

You're told how $\mathbf{P}$ relates to $\mathbf{E}$; the question states $$\mathbf{P} = \epsilon_0(\chi_1 + \chi_3E^2)\mathbf{E}.$$ The definition $$\mathbf{D} = \epsilon_0\mathbf{E} + \mathbf{P}$$ does not assume a linear relationship between $\mathbf{P}$ and $\mathbf{E}$.

 

[milkism]

You're told how $\mathbf{P}$ relates to $\mathbf{E}$; the question states $$\mathbf{P} = \epsilon_0(\chi_1 + \chi_3E^2)\mathbf{E}.$$ The definition $$\mathbf{D} = \epsilon_0\mathbf{E} + \mathbf{P}$$ does not assume a linear relationship between $\mathbf{P}$ and $\mathbf{E}$.

So basically $$\mathbf E = \frac{\mathbf P}{\epsilon_0 \chi_1 + \chi_3 E^2}$$
and
$$\mathbf D= \mathbf P \left( \frac{1}{ \chi_1 + \chi_3 E^2} + 1 \right)$$
The question doesn't really clarify what the fields should be on terms of what. Would you do what I did also? (Have no idea why it won't latex.)

Mentor (@Mark44) note: I fixed the LaTeX. Please let me know if it's what you intended.

 

[pasmith]

You are told to calculate the fields between the plates. Start with $\mathbf{E}$. You are asked to assume that the plates are infinite. Do you know how to find the field between a pair of infinite flat plates a fixed distance apart with a prescribed potential difference between them?

 

[milkism]

You are told to calculate the fields between the plates. Start with $\mathbf{E}$. You are asked to assume that the plates are infinite. Do you know how to find the field between a pair of infinite flat plates a fixed distance apart with a prescribed potential difference between them?

Does V/d still apply when there's a dielectric between?

 

[milkism]

So basically $$\mathbf E = \frac{\mathbf P}{\epsilon_0 \chi_1 + \chi_3 \mathbf E^2}$$
and
$$\mathbf D= \mathbf P \left( \frac{1}{ \chi_1 + \chi_3 \mathbf E^2} + 1 \right)$$
The question doesn't really clarify what the fields should be on terms of what. Would you do what I did also? (Have no idea why it won't latex.)

Mentor (@Mark44) note: I fixed the LaTeX. Please let me know if it's what you intended.

Yes, thank you, but E shouldn't be vectored, we don't want to divide vectors by vectors .

 

[milkism]

This is my new solution:

Are these correct?

 

[TSny]

From the relation $\mathbf{P} = \epsilon_0\left( \chi_1 + \chi_3 E^2 \right) \mathbf E$, shouldn't $\mathbf P$ have the same direction as $\mathbf E$?

You say that "$\mathbf P$ goes from negative to positive". Can you elaborate on this? Which positive and negative charges are you referring to here?

 

[milkism]

From the relation $\mathbf{P} = \epsilon_0\left( \chi_1 + \chi_3 E^2 \right) \mathbf E$, shouldn't $\mathbf P$ have the same direction as $\mathbf E$?

You say that "$\mathbf P$ goes from negative to positive". Can you elaborate on this? Which positive and negative charges are you referring to here?

Doesn't polarisation go from negative charge to positive charge, whereas electric field goes from positive to negative?

 

[milkism]

Doesn't polarisation go from negative charge to positive charge, whereas electric field goes from positive to negative?

Omg I mixed up direction of dipole moment with direction of polarisation, sorry.

 

[berkeman]

Yes, thank you, but E shouldn't be vectored, we don't want to divide vectors by vectors .

Fixed.