- #1
typeinnocent
- 14
- 0
Homework Statement
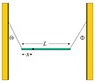
The bar is of nonuniform density so one end is heavier than the other. It has an overall length of 6.9 meters and is held in place by two very light wires. The left cord makes an angle with the wall of Θ = 39 degrees and the right cord makes an angle of Φ = 52 degrees.
Homework Equations
Net torque must equal zero, and the forces in the x and y direction must each equal zero.
The Attempt at a Solution
For net torque:
T2(cos52)(6.9-x)-T1(cos39)(x)-mg(3.45-x)=0 (with m being the mass of the bar)
For net force in the x direction:
T2(sin52) = T1(sin39)
For net force in the y direction:
T1(cos39)+T2(cos52) = mg
My pivot point was the center of mass, shown by the dot on the picture. Using these equations, I let T2 = T1(sin39/sin52). Then I substituted what mg equals into the net torque equation, and also substituted what T2 equals into the net torque equation. All of the T1's cancel out, and I'm able to solve for x. But the answer is incorrect. Any suggestions as to what I'm doing wrong?
Attachments
Last edited: